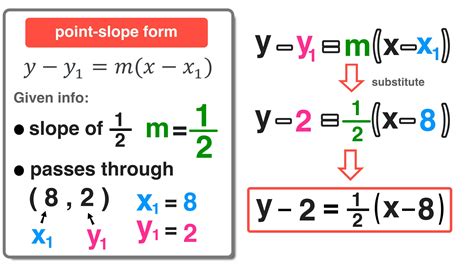
Point-slope form is a fundamental concept in algebra and geometry, used to represent linear equations in a unique and useful way. Unlike the slope-intercept form, which focuses on the slope and the y-intercept, the point-slope form emphasizes a specific point on the line and the slope. The formula for the point-slope form is (y - y_1 = m(x - x_1)), where (m) is the slope of the line, and ((x_1, y_1)) is a point on the line. This form is particularly useful for finding the equation of a line when you know one point on the line and the slope.
Here, we'll explore five examples that demonstrate how to apply the point-slope form in various scenarios.
Example 1: Finding the Equation of a Line Given a Point and Slope

Suppose you are given a point ((2, 3)) and a slope (m = 2). To find the equation of the line using the point-slope form, you substitute the given values into the formula.
Given: ((x_1, y_1) = (2, 3)) and (m = 2)
[y - y_1 = m(x - x_1)] [y - 3 = 2(x - 2)] [y - 3 = 2x - 4] [y = 2x - 1]
This is the equation of the line in slope-intercept form, but the process started with the point-slope form.
Example 2: Using Point-Slope Form to Show Parallel Lines

Two lines are parallel if they have the same slope but different y-intercepts. Given two points and their respective slopes, we can show whether two lines are parallel using the point-slope form.
Suppose we have Line A with a point ((1, 2)) and slope (m_A = 3), and Line B with a point ((3, 4)) and slope (m_B = 3).
For Line A: (y - 2 = 3(x - 1))
For Line B: (y - 4 = 3(x - 3))
Both equations show the same slope ((m = 3)), indicating that these lines are parallel.
Example 3: Finding the Slope of a Line Given Two Points

Sometimes, you might be given two points on a line and need to find the slope. The formula for slope is (m = \frac{y_2 - y_1}{x_2 - x_1}). After finding the slope, you can use one of the points and the slope to write the equation of the line in point-slope form.
Given points ((4, 5)) and ((6, 7)).
[m = \frac{7 - 5}{6 - 4} = \frac{2}{2} = 1]
Using point-slope form with one of the points, say ((4, 5)), and the slope (m = 1):
[y - 5 = 1(x - 4)] [y - 5 = x - 4] [y = x + 1]
Example 4: Converting from Standard Form to Point-Slope Form

Standard form of a line is (Ax + By = C). To convert this into point-slope form, you need to solve for (y) and then substitute a known point (which can be found by choosing an (x) value and solving for (y)) and the slope into the point-slope formula.
Given the line in standard form: (3x + 2y = 7)
Solving for (y): (2y = -3x + 7), thus (y = -\frac{3}{2}x + \frac{7}{2})
Slope (m = -\frac{3}{2}). To find a point on the line, let's use (x = 0), which gives (y = \frac{7}{2}).
So, a point on the line is ((0, \frac{7}{2})).
Using point-slope form: (y - \frac{7}{2} = -\frac{3}{2}(x - 0))
Example 5: Real-World Application

In real-world scenarios, such as in economics, physics, or engineering, linear equations can model various phenomena. For instance, suppose the cost (C) of producing (x) units of a product is given by a linear equation. If the cost of producing 10 units is $100 and the cost increases by $5 for each additional unit produced, you can model this situation using the point-slope form.
Given: Point ((10, 100)) and slope (m = 5)
[C - 100 = 5(x - 10)] [C - 100 = 5x - 50] [C = 5x + 50]
This equation represents the total cost (C) of producing (x) units.
These examples illustrate how versatile the point-slope form can be in solving problems related to linear equations. Whether you're working with given points and slopes, finding equations of lines, or applying linear models to real-world situations, understanding how to use the point-slope form effectively is a valuable skill.
Engagement: Have you encountered a situation where the point-slope form helped you solve a problem more easily? Share your experience or any questions you have about applying this form in the comments below.