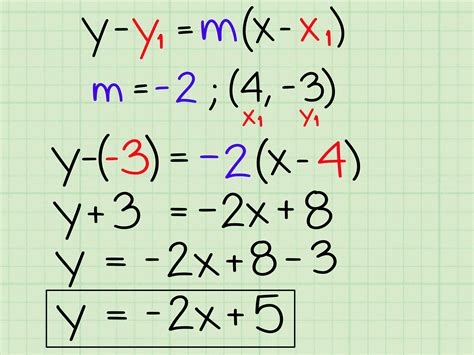Parallel lines are a fundamental concept in geometry and algebra, and understanding them is essential for solving various math problems. In this article, we will delve into the world of parallel lines in slope-intercept form, exploring what they are, how to identify them, and how to work with them.
What are Parallel Lines?

Parallel lines are two or more lines that lie in the same plane and never intersect, no matter how far they are extended. In other words, parallel lines have the same slope, but they are not the same line. This concept is crucial in mathematics, physics, engineering, and architecture, as it helps us understand the relationships between objects and their movements.
Understanding Slope-Intercept Form
To work with parallel lines, we need to understand the slope-intercept form of a linear equation. The slope-intercept form is a way of expressing a linear equation in the form y = mx + b, where:
- m is the slope of the line (a measure of how steep it is)
- b is the y-intercept (the point where the line crosses the y-axis)
For example, the equation y = 2x + 3 is in slope-intercept form, where the slope (m) is 2 and the y-intercept (b) is 3.
Identifying Parallel Lines in Slope-Intercept Form

To identify parallel lines in slope-intercept form, we need to look for lines with the same slope (m) but different y-intercepts (b). For instance:
- Line 1: y = 2x + 3
- Line 2: y = 2x + 5
Both lines have the same slope (m = 2), but they have different y-intercepts (b = 3 and b = 5). This means that these lines are parallel.
How to Determine if Two Lines are Parallel
To determine if two lines are parallel, follow these steps:
- Write both equations in slope-intercept form (y = mx + b).
- Compare the slopes (m) of both lines. If they are equal, the lines are parallel.
- Compare the y-intercepts (b) of both lines. If they are different, the lines are parallel.
Working with Parallel Lines

When working with parallel lines, there are several key concepts to keep in mind:
- Equal slopes: Parallel lines have the same slope, which means they rise or fall at the same rate.
- Different y-intercepts: Parallel lines have different y-intercepts, which means they cross the y-axis at different points.
- No intersection: Parallel lines never intersect, no matter how far they are extended.
These concepts are essential for solving problems involving parallel lines, such as finding the equation of a line parallel to a given line, or determining if two lines are parallel.
Real-World Applications of Parallel Lines
Parallel lines have numerous real-world applications, including:
- Architecture: Parallel lines are used to design buildings, bridges, and other structures.
- Engineering: Parallel lines are used to model the movement of objects, such as cars and trains.
- Physics: Parallel lines are used to describe the motion of particles and objects.
In conclusion, parallel lines in slope-intercept form are a fundamental concept in mathematics and have numerous real-world applications. By understanding the concept of parallel lines, you can solve a wide range of problems and appreciate the beauty of mathematics in the world around us.
What is the definition of parallel lines?
+Parallel lines are two or more lines that lie in the same plane and never intersect, no matter how far they are extended.
How do I identify parallel lines in slope-intercept form?
+To identify parallel lines in slope-intercept form, look for lines with the same slope (m) but different y-intercepts (b).
What are some real-world applications of parallel lines?
+Parallel lines have numerous real-world applications, including architecture, engineering, and physics.
We hope this article has helped you understand parallel lines in slope-intercept form. If you have any questions or comments, please feel free to share them below.