Standard form in math is a fundamental concept that has far-reaching implications in various mathematical disciplines, including algebra, geometry, and calculus. Despite its importance, many students struggle to grasp the underlying principles of standard form, leading to confusion and frustration. In this article, we will delve into the world of standard form, exploring its definition, significance, and applications in mathematics.
Mathematics is a language that relies heavily on notation and representation. Standard form is a specific way of expressing mathematical concepts, making it easier to communicate and manipulate mathematical objects. It provides a universal framework for representing numbers, equations, and functions, facilitating calculations, comparisons, and analyses.
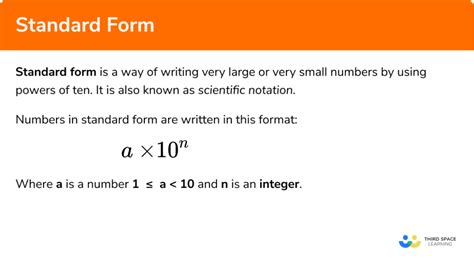
What is Standard Form in Math?

Standard form in math refers to a specific notation or representation of mathematical objects, such as numbers, equations, and functions. It is a way of expressing mathematical concepts in a unique and consistent manner, making it easier to understand, compare, and manipulate them. The standard form of a mathematical object is often denoted by a specific notation or symbol, which serves as a universal identifier.
For example, the standard form of a linear equation is ax + by = c, where a, b, and c are constants, and x and y are variables. This notation provides a clear and concise way of representing linear equations, making it easier to solve and compare them.
Types of Standard Forms in Math
There are several types of standard forms in math, each with its own notation and significance. Some of the most common types of standard forms include:
- Standard form of a linear equation: ax + by = c
- Standard form of a quadratic equation: ax^2 + bx + c = 0
- Standard form of a polynomial: a_nx^n + a_(n-1)x^(n-1) +... + a_1x + a_0
- Standard form of a rational function: f(x) = p(x)/q(x), where p(x) and q(x) are polynomials
Each of these standard forms has its own unique notation and significance, making it easier to communicate and manipulate mathematical concepts.
Importance of Standard Form in Math

Standard form in math is essential for several reasons:
- Clarity and precision: Standard form provides a clear and concise way of representing mathematical concepts, reducing ambiguity and confusion.
- Universality: Standard form is a universal language, making it easier to communicate mathematical ideas across different cultures and disciplines.
- Comparability: Standard form enables comparison and analysis of mathematical objects, facilitating calculations and problem-solving.
- Manipulation: Standard form provides a framework for manipulating mathematical objects, making it easier to solve equations, simplify expressions, and perform calculations.
Applications of Standard Form in Math
Standard form in math has numerous applications across various mathematical disciplines, including:
- Algebra: Standard form is used to solve linear and quadratic equations, manipulate polynomials, and analyze rational functions.
- Geometry: Standard form is used to represent geometric shapes, such as lines, circles, and conic sections.
- Calculus: Standard form is used to represent functions, derivatives, and integrals, facilitating calculations and problem-solving.
How to Work with Standard Form in Math

Working with standard form in math requires a deep understanding of mathematical concepts and notation. Here are some tips for working with standard form:
- Understand the notation: Familiarize yourself with the notation and symbols used to represent mathematical objects in standard form.
- Practice, practice, practice: Practice converting mathematical objects to standard form and manipulating them using algebraic operations.
- Use online resources: Utilize online resources, such as calculators and math software, to help with calculations and problem-solving.
- Seek help when needed: Don't hesitate to seek help from teachers, tutors, or online resources when struggling with standard form.
Common Mistakes to Avoid
When working with standard form in math, it's essential to avoid common mistakes, such as:
- Incorrect notation: Using incorrect notation or symbols can lead to confusion and errors.
- Insufficient simplification: Failing to simplify expressions or equations can make them difficult to work with.
- Inconsistent application: Applying standard form inconsistently can lead to errors and confusion.
By avoiding these common mistakes, you can ensure that you're working with standard form effectively and efficiently.
Conclusion
Standard form in math is a fundamental concept that provides a clear and concise way of representing mathematical objects. Its significance extends across various mathematical disciplines, making it an essential tool for problem-solving and communication. By understanding the definition, importance, and applications of standard form, you can improve your math skills and become more proficient in manipulating mathematical objects.
We hope this article has provided you with a comprehensive understanding of standard form in math. If you have any questions or comments, please feel free to share them below.
What is the standard form of a linear equation?
+The standard form of a linear equation is ax + by = c, where a, b, and c are constants, and x and y are variables.
Why is standard form important in math?
+Standard form is important in math because it provides a clear and concise way of representing mathematical objects, making it easier to communicate and manipulate them.
How do I convert a mathematical object to standard form?
+To convert a mathematical object to standard form, you need to understand the notation and symbols used to represent it. Practice converting mathematical objects to standard form and manipulating them using algebraic operations.