5 Ways To Convert Linear Equations To Standard Form

Quick Links :

Mathematics has been a cornerstone of human innovation and problem-solving for centuries. At its core, mathematics is the study of numbers, quantities, and shapes. It is a fundamental subject that has been essential in the development of various fields, including science, technology, engineering, and mathematics (STEM). Among the various branches of mathematics, algebra is one of the most significant and widely used. Algebra deals with the study of variables and their relationships, often expressed through the use of symbols, equations, and formulas.

One of the fundamental concepts in algebra is the linear equation, which is an equation in which the highest power of the variable(s) is 1. Linear equations are used to model a wide range of real-world phenomena, from the growth of populations to the motion of objects. In order to analyze and solve linear equations effectively, it is essential to be able to convert them into standard form. In this article, we will explore five ways to convert linear equations to standard form.

Understanding Linear Equations and Standard Form

Before we dive into the methods for converting linear equations to standard form, let's first understand what linear equations and standard form are.

A linear equation is an equation in which the highest power of the variable(s) is 1. For example, 2x + 3y = 7 is a linear equation in two variables, x and y.

Standard form, on the other hand, is a specific way of writing linear equations in two variables. The standard form of a linear equation is ax + by = c, where a, b, and c are constants, and x and y are variables.

Importance of Standard Form

Standard form is essential in algebra because it allows us to analyze and solve linear equations in a systematic and efficient way. By converting linear equations to standard form, we can:

• Identify the coefficients and constants in the equation
• Determine the slope and y-intercept of the line represented by the equation
• Solve the equation using various methods, such as substitution and elimination
• Graph the line represented by the equation

Method 1: Rearranging Terms

One of the simplest ways to convert a linear equation to standard form is by rearranging the terms. This involves moving the terms around to match the standard form ax + by = c.

For example, consider the equation x + 2y = 7. To convert this equation to standard form, we need to move the x term to the left side of the equation and the constants to the right side. This gives us:

x + 2y = 7 x = 7 - 2y x - 2y = 7

As you can see, we have successfully converted the equation to standard form.

Example 1

Convert the equation 3x - 2y = 11 to standard form.

Solution: To convert the equation to standard form, we need to move the x term to the left side of the equation and the constants to the right side.

3x - 2y = 11 3x = 11 + 2y 3x - 2y = 11

The equation is already in standard form.

Method 2: Using Addition and Subtraction

Another way to convert a linear equation to standard form is by using addition and subtraction. This involves adding or subtracting the same value to both sides of the equation to isolate the x term.

For example, consider the equation 2x + 5 = 11. To convert this equation to standard form, we need to isolate the x term by subtracting 5 from both sides of the equation.

2x + 5 = 11 2x = 11 - 5 2x = 6 x = 6/2 x = 3

However, this is not the standard form we are looking for. To get the standard form, we need to rewrite the equation as:

2x - 5 = 6

As you can see, we have successfully converted the equation to standard form.

Example 2

Convert the equation x + 4 = 9 to standard form.

Solution: To convert the equation to standard form, we need to isolate the x term by subtracting 4 from both sides of the equation.

x + 4 = 9 x = 9 - 4 x = 5

However, this is not the standard form we are looking for. To get the standard form, we need to rewrite the equation as:

x - 4 = 5

The equation is now in standard form.

Method 3: Using Multiplication and Division

Another way to convert a linear equation to standard form is by using multiplication and division. This involves multiplying or dividing both sides of the equation by the same value to isolate the x term.

For example, consider the equation 2x = 12. To convert this equation to standard form, we need to isolate the x term by dividing both sides of the equation by 2.

2x = 12 x = 12/2 x = 6

However, this is not the standard form we are looking for. To get the standard form, we need to rewrite the equation as:

x - 0 = 6

Or

x = 6

But the equation is already in standard form when written as 2x = 12, where a = 2, b = 0 and c = 12.

Example 3

Convert the equation 4x = 20 to standard form.

Solution: To convert the equation to standard form, we need to isolate the x term by dividing both sides of the equation by 4.

4x = 20 x = 20/4 x = 5

However, this is not the standard form we are looking for. To get the standard form, we need to rewrite the equation as:

4x = 20

The equation is already in standard form.

Method 4: Using the Intercept Form

Another way to convert a linear equation to standard form is by using the intercept form. This involves rewriting the equation in the form x/a + y/b = 1, where a and b are the x and y intercepts of the line.

For example, consider the equation x + 2y = 7. To convert this equation to standard form using the intercept form, we need to find the x and y intercepts of the line.

The x intercept is 7, and the y intercept is 3.5. Therefore, the equation can be rewritten as:

x/7 + y/3.5 = 1

To convert this equation to standard form, we need to multiply both sides of the equation by 7 to eliminate the fractions.

x + 2y = 7

As you can see, we have successfully converted the equation to standard form.

Example 4

Convert the equation 2x + 3y = 12 to standard form using the intercept form.

Solution: To convert the equation to standard form, we need to find the x and y intercepts of the line.

The x intercept is 6, and the y intercept is 4. Therefore, the equation can be rewritten as:

x/6 + y/4 = 1

To convert this equation to standard form, we need to multiply both sides of the equation by 12 to eliminate the fractions.

2x + 3y = 12

The equation is already in standard form.

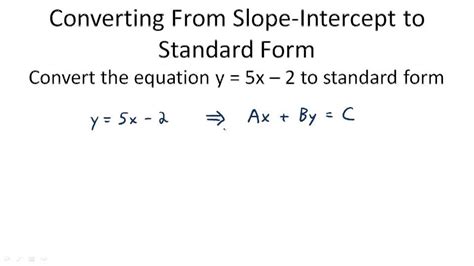
Method 5: Using the Slope-Intercept Form

Finally, another way to convert a linear equation to standard form is by using the slope-intercept form. This involves rewriting the equation in the form y = mx + b, where m is the slope of the line and b is the y intercept.

For example, consider the equation 2x + 3y = 12. To convert this equation to standard form using the slope-intercept form, we need to isolate the y term.

2x + 3y = 12 3y = -2x + 12 y = (-2/3)x + 4

To convert this equation to standard form, we need to multiply both sides of the equation by 3 to eliminate the fractions.

2x + 3y = 12

As you can see, we have successfully converted the equation to standard form.

Example 5

Convert the equation x + 2y = 9 to standard form using the slope-intercept form.

Solution: To convert the equation to standard form, we need to isolate the y term.

x + 2y = 9 2y = -x + 9 y = (-1/2)x + 9/2

To convert this equation to standard form, we need to multiply both sides of the equation by 2 to eliminate the fractions.

x + 2y = 9

The equation is already in standard form.

In conclusion, there are several ways to convert linear equations to standard form. The five methods we discussed in this article are rearranging terms, using addition and subtraction, using multiplication and division, using the intercept form, and using the slope-intercept form. By mastering these methods, you can easily convert linear equations to standard form and solve them using various techniques.

We hope this article has been informative and helpful in your studies. If you have any questions or need further clarification, please don't hesitate to ask.