Alternate Forms Of A Derivative Explained

Quick Links :

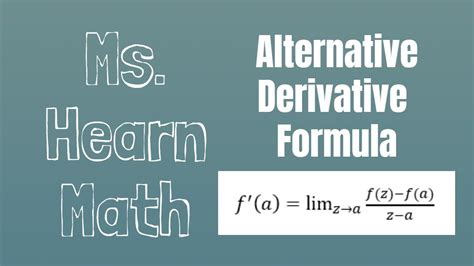
The concept of a derivative is a fundamental aspect of calculus and is used to measure the rate of change of a function. While the traditional definition of a derivative is well-known, there are alternative forms of a derivative that can be used in different contexts. These alternative forms can provide new insights and perspectives on the behavior of functions, and can be used to solve problems that cannot be solved using the traditional definition.

Introduction to Alternate Forms of Derivatives

In mathematics, the derivative of a function is defined as the limit of the ratio of the change in the output value to the change in the input value, as the input value approaches zero. This definition is known as the limit definition of a derivative, and it is widely used in calculus and other branches of mathematics. However, there are other forms of derivatives that can be used in different contexts, such as the logarithmic derivative, the reciprocal derivative, and the implicit derivative.

Logarithmic Derivative

The logarithmic derivative is a type of derivative that is used to study the behavior of functions that involve logarithms. It is defined as the derivative of the logarithm of a function, and it is used to solve problems that involve logarithmic functions. The logarithmic derivative is particularly useful when studying the behavior of functions that involve exponential growth or decay.

For example, consider the function f(x) = e^x. The logarithmic derivative of this function is f'(x) = 1/x, which is the reciprocal of the function. This result can be used to solve problems that involve exponential growth or decay, such as population growth or radioactive decay.

Reciprocal Derivative

The reciprocal derivative is a type of derivative that is used to study the behavior of functions that involve reciprocals. It is defined as the derivative of the reciprocal of a function, and it is used to solve problems that involve reciprocal functions. The reciprocal derivative is particularly useful when studying the behavior of functions that involve inverse relationships, such as the relationship between the radius and the area of a circle.

For example, consider the function f(x) = 1/x. The reciprocal derivative of this function is f'(x) = -1/x^2, which is the negative of the reciprocal of the function. This result can be used to solve problems that involve inverse relationships, such as the relationship between the radius and the area of a circle.

Implicit Derivative

The implicit derivative is a type of derivative that is used to study the behavior of functions that involve implicit relationships. It is defined as the derivative of an implicit function, and it is used to solve problems that involve implicit functions. The implicit derivative is particularly useful when studying the behavior of functions that involve implicit relationships, such as the relationship between the variables in a system of equations.

For example, consider the equation x^2 + y^2 = 1. The implicit derivative of this equation is dy/dx = -x/y, which is the negative of the ratio of the variables. This result can be used to solve problems that involve implicit relationships, such as the relationship between the variables in a system of equations.

Benefits of Alternate Forms of Derivatives

The alternate forms of derivatives have several benefits that make them useful in different contexts. One of the main benefits is that they provide new insights and perspectives on the behavior of functions. By using different forms of derivatives, mathematicians and scientists can gain a deeper understanding of the behavior of functions and solve problems that cannot be solved using the traditional definition.

Another benefit of alternate forms of derivatives is that they can be used to solve problems that involve special functions, such as logarithmic functions or reciprocal functions. These functions are commonly used in mathematics and science, and the alternate forms of derivatives provide a powerful tool for studying their behavior.

Finally, the alternate forms of derivatives can be used to develop new mathematical models and theories. By using different forms of derivatives, mathematicians and scientists can develop new models and theories that can be used to solve complex problems in mathematics and science.

Applications of Alternate Forms of Derivatives

The alternate forms of derivatives have several applications in mathematics and science. One of the main applications is in the study of special functions, such as logarithmic functions or reciprocal functions. These functions are commonly used in mathematics and science, and the alternate forms of derivatives provide a powerful tool for studying their behavior.

Another application of alternate forms of derivatives is in the development of new mathematical models and theories. By using different forms of derivatives, mathematicians and scientists can develop new models and theories that can be used to solve complex problems in mathematics and science.

Finally, the alternate forms of derivatives can be used in engineering and physics to solve problems that involve complex systems and relationships. For example, the implicit derivative can be used to study the behavior of complex systems, such as electrical circuits or mechanical systems.

Conclusion

In conclusion, the alternate forms of derivatives provide a powerful tool for studying the behavior of functions and solving problems in mathematics and science. By using different forms of derivatives, mathematicians and scientists can gain a deeper understanding of the behavior of functions and develop new mathematical models and theories.

We hope this article has provided you with a comprehensive understanding of the alternate forms of derivatives and their applications. If you have any questions or comments, please feel free to ask.