Master The Intercept Form Of A Quadratic In 5 Steps

Quick Links :

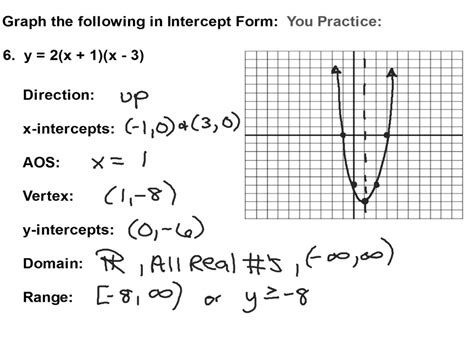
Quadratic equations are a fundamental concept in algebra, and understanding their various forms is crucial for solving problems in mathematics, physics, and engineering. One of the most useful forms of a quadratic equation is the intercept form, also known as the factored form or the x-intercept form. In this article, we will explore the intercept form of a quadratic equation, its significance, and provide a step-by-step guide on how to master it in 5 easy steps.

A quadratic equation in the intercept form is expressed as y = a(x - p)(x - q), where p and q are the x-intercepts of the parabola. The x-intercepts are the points where the parabola intersects the x-axis, and they play a crucial role in understanding the behavior of the quadratic function. The intercept form is particularly useful when finding the roots of a quadratic equation, identifying the x-intercepts, and sketching the graph of the parabola.

Step 1: Understand the Basics of Quadratic Equations

Before diving into the intercept form, it's essential to have a solid understanding of the basics of quadratic equations. A quadratic equation is a polynomial equation of degree two, which means the highest power of the variable (usually x) is two. The general form of a quadratic equation is ax^2 + bx + c = 0, where a, b, and c are constants. Quadratic equations can be solved using various methods, including factoring, the quadratic formula, and graphing.

Key Concepts:

• Quadratic equations are polynomial equations of degree two.
• The general form of a quadratic equation is ax^2 + bx + c = 0.
• Quadratic equations can be solved using factoring, the quadratic formula, and graphing.

Step 2: Learn the Intercept Form Formula

The intercept form of a quadratic equation is expressed as y = a(x - p)(x - q), where p and q are the x-intercepts of the parabola. This form is particularly useful when finding the roots of a quadratic equation and identifying the x-intercepts. To master the intercept form, it's essential to understand the formula and how to apply it.

Key Concepts:

• The intercept form of a quadratic equation is y = a(x - p)(x - q).
• p and q are the x-intercepts of the parabola.
• The intercept form is useful for finding the roots of a quadratic equation and identifying the x-intercepts.

Step 3: Practice Converting Quadratic Equations to Intercept Form

Converting a quadratic equation to intercept form involves factoring the equation and identifying the x-intercepts. To master this step, practice converting different quadratic equations to intercept form. Start with simple equations and gradually move on to more complex ones.

Key Concepts:

• Converting a quadratic equation to intercept form involves factoring and identifying the x-intercepts.
• Practice converting different quadratic equations to intercept form.
• Start with simple equations and gradually move on to more complex ones.

Step 4: Learn to Identify the X-Intercepts

Identifying the x-intercepts is a crucial step in mastering the intercept form of a quadratic equation. The x-intercepts are the points where the parabola intersects the x-axis, and they can be found by factoring the equation or using the quadratic formula.

Key Concepts:

• The x-intercepts are the points where the parabola intersects the x-axis.
• The x-intercepts can be found by factoring the equation or using the quadratic formula.
• Identifying the x-intercepts is essential for understanding the behavior of the quadratic function.

Step 5: Apply the Intercept Form to Real-World Problems

The intercept form of a quadratic equation has numerous applications in real-world problems, such as physics, engineering, and economics. By mastering the intercept form, you can solve complex problems involving quadratic equations and model real-world phenomena.

Key Concepts:

• The intercept form of a quadratic equation has numerous applications in real-world problems.
• Mastering the intercept form enables you to solve complex problems involving quadratic equations.
• The intercept form can be used to model real-world phenomena in physics, engineering, and economics.

By following these 5 steps, you can master the intercept form of a quadratic equation and improve your problem-solving skills in mathematics and other fields. Remember to practice regularly and apply the intercept form to real-world problems to reinforce your understanding.

Now that you've reached the end of this article, we'd love to hear from you! What are some of the most challenging quadratic equations you've encountered? How do you think the intercept form can be applied to real-world problems? Share your thoughts and questions in the comments section below!