Radicals can be a challenging concept to grasp in mathematics, especially for those who are new to algebra. However, simplifying radicals can be made easier by following a series of steps. In this article, we will explore the 5 steps to simplify radicals, making it easier for you to understand and work with these mathematical expressions.
Radicals are an essential part of algebra, and they can be found in various mathematical operations, such as addition, subtraction, multiplication, and division. Simplifying radicals can help you to better understand the underlying mathematical concepts and to solve problems more efficiently.

Step 1: Identify the Radical
The first step in simplifying radicals is to identify the radical expression. A radical expression is any mathematical expression that contains a radical sign (√). The radical sign is used to indicate the nth root of a number. For example, √x represents the square root of x, while ³√x represents the cube root of x.
When identifying the radical expression, make sure to note the index (the number outside the radical sign) and the radicand (the number inside the radical sign). The index tells you which root to take, while the radicand is the number that you are taking the root of.

Step 2: Look for Perfect Squares or Perfect Cubes
The next step is to look for perfect squares or perfect cubes within the radicand. A perfect square is a number that can be expressed as the square of an integer, such as 4 (which is 2²) or 9 (which is 3²). A perfect cube is a number that can be expressed as the cube of an integer, such as 8 (which is 2³) or 27 (which is 3³).
If you find a perfect square or perfect cube within the radicand, you can simplify the radical expression by taking the square or cube root of that number. For example, √4 can be simplified to 2, since 4 is a perfect square.

Step 3: Simplify the Radical
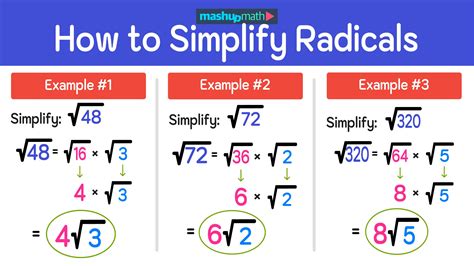
Once you have identified any perfect squares or perfect cubes within the radicand, you can simplify the radical expression. To simplify the radical, divide the radicand by the perfect square or perfect cube, and then take the square or cube root of the result.
For example, √12 can be simplified by dividing 12 by 4 (which is a perfect square), resulting in √3. Similarly, ³√48 can be simplified by dividing 48 by 8 (which is a perfect cube), resulting in ³√6.

Step 4: Rationalize the Denominator
If the radical expression has a denominator (i.e., it is a fraction), you may need to rationalize the denominator. Rationalizing the denominator involves multiplying the numerator and denominator by a radical expression that will eliminate the radical in the denominator.
For example, √2/√3 can be rationalized by multiplying the numerator and denominator by √3, resulting in √6/3.

Step 5: Check Your Work
The final step is to check your work. Make sure that you have simplified the radical expression correctly and that you have not made any errors.
It is also a good idea to check your work by plugging the simplified radical expression back into the original equation to ensure that it is true.

In conclusion, simplifying radicals can be made easier by following these 5 steps. By identifying the radical expression, looking for perfect squares or perfect cubes, simplifying the radical, rationalizing the denominator, and checking your work, you can simplify even the most complex radical expressions.
We hope that this article has been helpful in explaining the 5 steps to simplify radicals. Do you have any questions or comments about simplifying radicals? Share them with us in the comments section below.
What is a radical expression?
+A radical expression is any mathematical expression that contains a radical sign (√). The radical sign is used to indicate the nth root of a number.
What is a perfect square or perfect cube?
+A perfect square is a number that can be expressed as the square of an integer, such as 4 (which is 2²) or 9 (which is 3²). A perfect cube is a number that can be expressed as the cube of an integer, such as 8 (which is 2³) or 27 (which is 3³).
How do I rationalize the denominator?
+Rationalizing the denominator involves multiplying the numerator and denominator by a radical expression that will eliminate the radical in the denominator.