Write In Radical Form: A Step-By-Step Guide

Quick Links :

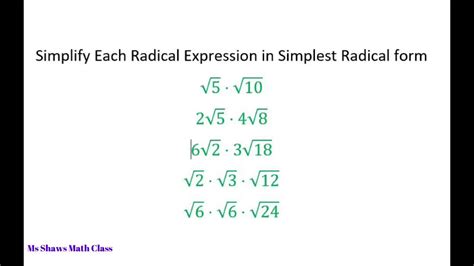
In today's fast-paced world, understanding and utilizing radicals in mathematics and science is crucial for solving complex problems and uncovering new discoveries. Radicals, also known as roots, play a significant role in various mathematical operations, from simple arithmetic to advanced calculus. In this comprehensive guide, we will delve into the world of radicals, exploring their properties, applications, and step-by-step procedures for working with them.

Understanding Radicals

A radical is a mathematical symbol that represents a root of a number. The most common radical is the square root, denoted by the symbol √. Radicals can be classified into two main categories: square roots and nth roots. Square roots are used to find the number that, when multiplied by itself, gives a specified value. Nth roots, on the other hand, are used to find the number that, when raised to the power of n, gives a specified value.

Properties of Radicals

Radicals have several important properties that are essential for working with them. These properties include:

• The product property: The product of two radicals is equal to the radical of the product of the two numbers. For example, √a × √b = √(a × b).
• The quotient property: The quotient of two radicals is equal to the radical of the quotient of the two numbers. For example, √a ÷ √b = √(a ÷ b).
• The power property: The power of a radical is equal to the radical of the power of the number. For example, (√a)^n = √(a^n).

Working with Radicals

Now that we have explored the properties of radicals, let's move on to the step-by-step procedures for working with them.

Simplifying Radicals

Simplifying radicals involves expressing the radical in its simplest form. To simplify a radical, we need to find the largest perfect square that divides the number inside the radical.

For example, √12 can be simplified as follows:

√12 = √(4 × 3) = √4 × √3 = 2√3

Adding and Subtracting Radicals

Adding and subtracting radicals involve combining like terms. Like terms are radicals that have the same number inside the radical.

For example:

√a + √a = 2√a

√a - √a = 0

Multiplying and Dividing Radicals

Multiplying and dividing radicals involve using the product and quotient properties.

For example:

√a × √b = √(a × b)

√a ÷ √b = √(a ÷ b)

Real-World Applications of Radicals

Radicals have numerous real-world applications in various fields, including physics, engineering, and computer science.

• Physics: Radicals are used to calculate distances, velocities, and accelerations in physics. For example, the distance traveled by an object can be calculated using the formula √(x^2 + y^2).
• Engineering: Radicals are used to design and optimize systems, such as electronic circuits and mechanical systems.
• Computer Science: Radicals are used in algorithms for solving complex problems, such as finding the shortest path in a graph.

In conclusion, radicals are an essential part of mathematics and science, with numerous real-world applications. By understanding the properties and step-by-step procedures for working with radicals, we can unlock new discoveries and solve complex problems.

Take the next step in your learning journey by exploring more advanced topics in mathematics and science. Share your thoughts and experiences with radicals in the comments section below.