5 Steps To Write Ellipse In Standard Form

Quick Links :

Hide

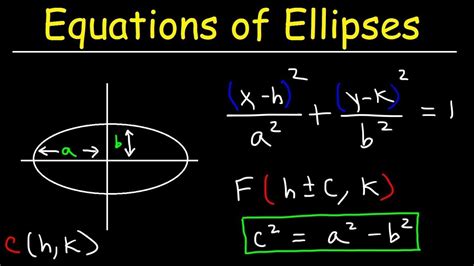
Writing an ellipse in standard form is a fundamental concept in mathematics, particularly in algebra and geometry. An ellipse is a closed curve on a plane surrounding two focal points such that the sum of the distances to the two focal points is constant. The standard form of an ellipse equation provides a concise way to describe its properties, including its center, major and minor axes, and the distance between the foci.

Understanding the Components of an Ellipse

Before diving into the steps to write an ellipse in standard form, it's essential to understand the key components of an ellipse:

• Major Axis: The longest diameter of the ellipse, which passes through the center and both foci.
• Minor Axis: The shortest diameter of the ellipse, which passes through the center and is perpendicular to the major axis.
• Center: The midpoint of the major axis, which is also the midpoint of the ellipse.
• Foci: Two fixed points inside the ellipse, equidistant from the center, that help define the ellipse's shape.
• Distance between Foci: The length between the two foci, which is also known as 2c.

Step 1: Identify the Center of the Ellipse

The first step in writing an ellipse in standard form is to identify its center. The center of the ellipse is usually denoted as (h, k), where h is the x-coordinate and k is the y-coordinate. If the ellipse is centered at the origin (0, 0), then h = 0 and k = 0.

Example:

Suppose we have an ellipse centered at (2, 3). In this case, h = 2 and k = 3.

Step 2: Determine the Lengths of the Major and Minor Axes

The lengths of the major and minor axes are crucial in defining the shape of the ellipse. The major axis is usually denoted as 2a, while the minor axis is denoted as 2b. If the major axis is horizontal, then the standard form of the ellipse equation will be in terms of x. If the major axis is vertical, then the standard form will be in terms of y.

Example:

Suppose we have an ellipse with a major axis of length 10 and a minor axis of length 6. In this case, a = 5 and b = 3.

Step 3: Calculate the Distance between the Foci

The distance between the foci, denoted as 2c, can be calculated using the formula: c = √(a^2 - b^2). This formula is derived from the Pythagorean theorem and is essential in defining the shape of the ellipse.

Example:

Using the values from the previous example, we can calculate the distance between the foci: c = √(5^2 - 3^2) = √(25 - 9) = √16 = 4.

Step 4: Write the Standard Form of the Ellipse Equation

Now that we have the necessary components, we can write the standard form of the ellipse equation. If the major axis is horizontal, the equation will be in the form: (x - h)^2/a^2 + (y - k)^2/b^2 = 1. If the major axis is vertical, the equation will be in the form: (x - h)^2/b^2 + (y - k)^2/a^2 = 1.

Example:

Using the values from the previous examples, we can write the standard form of the ellipse equation: (x - 2)^2/5^2 + (y - 3)^2/3^2 = 1.

Step 5: Verify the Ellipse Equation

The final step is to verify the ellipse equation by checking its properties. We can do this by graphing the ellipse and checking its shape, center, and axes.

Example:

By graphing the ellipse equation (x - 2)^2/5^2 + (y - 3)^2/3^2 = 1, we can verify that it has the correct shape, center, and axes.

By following these five steps, we can write an ellipse in standard form and understand its properties.

Invitation to Engage: If you have any questions or need further clarification on writing an ellipse in standard form, please leave a comment below. Additionally, feel free to share this article with others who may benefit from it.