Write A Parabola Equation In Standard Form Easily

Quick Links :

Writing a parabola equation in standard form can seem daunting, but with the right approach, it can be a straightforward process. In this article, we will explore the world of parabolas, understand their importance, and learn how to write a parabola equation in standard form with ease.

What is a Parabola?

A parabola is a type of quadratic equation that represents a U-shaped curve. It is a fundamental concept in algebra and is used to model various real-world phenomena, such as the trajectory of a projectile, the shape of a satellite dish, and the path of a roller coaster.

Why is it Important to Write a Parabola Equation in Standard Form?

Writing a parabola equation in standard form is essential because it allows us to easily identify the key features of the parabola, such as its vertex, axis of symmetry, and direction of opening. This information is crucial in various fields, including physics, engineering, and economics.

What is the Standard Form of a Parabola Equation?

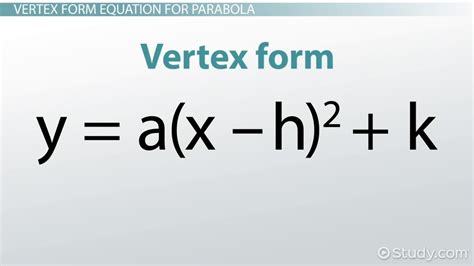
The standard form of a parabola equation is:

y = a(x - h)^2 + k

where:

• (h, k) is the vertex of the parabola
• a is the coefficient that determines the direction and width of the parabola
• x is the independent variable
• y is the dependent variable

How to Write a Parabola Equation in Standard Form

To write a parabola equation in standard form, follow these steps:

1. Identify the vertex of the parabola.
2. Determine the coefficient "a" that determines the direction and width of the parabola.
3. Write the equation in the standard form y = a(x - h)^2 + k.

Examples of Writing Parabola Equations in Standard Form

Let's consider a few examples:

• Example 1: Write the equation of a parabola with vertex (2, 3) and coefficient a = 2. y = 2(x - 2)^2 + 3

• Example 2: Write the equation of a parabola with vertex (-1, 4) and coefficient a = -3. y = -3(x + 1)^2 + 4

• Example 3: Write the equation of a parabola with vertex (0, 0) and coefficient a = 1. y = x^2

Benefits of Writing Parabola Equations in Standard Form

Writing parabola equations in standard form has several benefits:

• Easy identification of key features, such as vertex and axis of symmetry
• Simplified graphing and analysis of the parabola
• Enhanced understanding of the relationship between the parabola and its equation

Common Mistakes to Avoid When Writing Parabola Equations in Standard Form

When writing parabola equations in standard form, it's essential to avoid common mistakes, such as:

• Incorrect identification of the vertex
• Incorrect determination of the coefficient "a"
• Failure to write the equation in the standard form

Conclusion

Writing a parabola equation in standard form is a crucial skill in algebra and has numerous applications in various fields. By understanding the importance of standard form and following the steps outlined in this article, you can easily write parabola equations in standard form and gain a deeper understanding of these fundamental curves.

We hope this article has been informative and helpful. If you have any questions or comments, please feel free to share them below.