3 Steps To Write Decimals In Expanded Form

Quick Links :

Decimals are a crucial part of mathematics, and understanding how to write them in expanded form is a fundamental skill that can benefit students and professionals alike. Expanded form, also known as expanded notation, is a way of expressing a number in terms of its individual digits and their place values. In this article, we will explore the steps to write decimals in expanded form, providing examples and explanations to help you master this essential skill.

Why Learn Expanded Form?

Learning expanded form can help you better understand the value of each digit in a decimal number. It can also assist you in performing mathematical operations, such as addition and subtraction, with greater accuracy. Moreover, expanded form can be used to compare and order decimal numbers, making it a valuable tool in various mathematical applications.

Step 1: Identify the Decimal Number

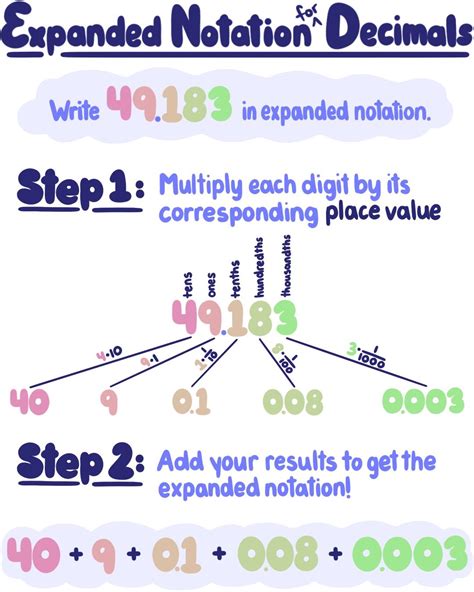
The first step in writing a decimal in expanded form is to identify the decimal number you want to work with. Let's take the decimal number 4.27 as an example. This number can be broken down into its individual digits, each with its own place value.

Step 2: Break Down the Decimal Number

To break down the decimal number 4.27, we can start by identifying the whole number part, which is 4. The decimal part is 0.27. We can further break down the decimal part into its individual digits, each with its own place value.

• 0.2 is in the tenths place
• 0.07 is in the hundredths place

Step 3: Write the Decimal Number in Expanded Form

Now that we have broken down the decimal number into its individual digits and place values, we can write it in expanded form. The expanded form of 4.27 is:

4 + 0.2 + 0.07

This can be read as "4 plus 2 tenths plus 7 hundredths."

Example Problems

Let's try some example problems to practice writing decimals in expanded form.

• Write the decimal number 3.45 in expanded form. Answer: 3 + 0.4 + 0.05
• Write the decimal number 2.91 in expanded form. Answer: 2 + 0.9 + 0.01

Benefits of Writing Decimals in Expanded Form

Writing decimals in expanded form can have several benefits, including:

• Improved understanding of place value
• Enhanced accuracy in mathematical operations
• Better comparison and ordering of decimal numbers

Real-World Applications

Writing decimals in expanded form has several real-world applications, including:

• Science: Scientists often use decimals to express measurements and quantities. Writing these decimals in expanded form can help them better understand the values they are working with.
• Finance: Financial calculations often involve decimals. Writing these decimals in expanded form can help professionals perform calculations with greater accuracy.
• Engineering: Engineers often use decimals to express measurements and quantities. Writing these decimals in expanded form can help them better understand the values they are working with.

Common Mistakes to Avoid

When writing decimals in expanded form, there are several common mistakes to avoid, including:

• Incorrectly identifying the place value of each digit
• Failing to include all digits in the expanded form
• Writing the digits in the wrong order

Conclusion

In conclusion, writing decimals in expanded form is a valuable skill that can benefit students and professionals alike. By following the three steps outlined in this article, you can master this skill and improve your understanding of decimal numbers.

We hope this article has been informative and helpful. If you have any questions or comments, please don't hesitate to share them with us.