5 Easy Steps To Convert Equations

Quick Links :

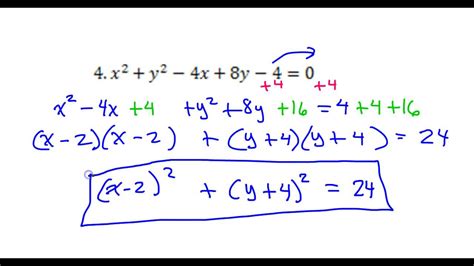
Converting equations from one form to another can be a daunting task, but with the right approach, it can be simplified and made more manageable. Equations are a fundamental concept in mathematics, physics, engineering, and other fields, and being able to convert them from one form to another is an essential skill. In this article, we will explore the importance of converting equations and provide a step-by-step guide on how to do it easily.

Converting equations is crucial in various applications, such as solving mathematical problems, modeling real-world phenomena, and optimizing systems. It can help simplify complex equations, reveal underlying patterns, and facilitate the solution of problems. Moreover, converting equations can also provide insights into the properties and behavior of the system being modeled.

In this article, we will discuss the benefits of converting equations, the different types of conversions, and provide a step-by-step guide on how to convert equations easily.

Understanding the Types of Equation Conversions

There are several types of equation conversions, including:

• Linear to Quadratic: Converting a linear equation to a quadratic equation involves introducing a squared term.
• Quadratic to Linear: Converting a quadratic equation to a linear equation involves factoring or completing the square.
• Polynomial to Rational: Converting a polynomial equation to a rational equation involves dividing by a polynomial.
• Rational to Polynomial: Converting a rational equation to a polynomial equation involves multiplying by a polynomial.

5 Easy Steps to Convert Equations

Converting equations can seem like a daunting task, but by following these easy steps, you can simplify the process and achieve the desired conversion.

Step 1: Identify the Type of Conversion

The first step is to identify the type of conversion needed. Determine the initial and final forms of the equation and the type of conversion required.

Step 2: Write Down the Initial Equation

Write down the initial equation in its original form. Make sure to include all variables and constants.

Step 3: Apply the Conversion Formula

Apply the conversion formula or technique to transform the initial equation into the desired form. This may involve factoring, completing the square, or dividing by a polynomial.

Step 4: Simplify the Equation

Simplify the resulting equation by combining like terms, canceling out common factors, and rearranging the terms.

Step 5: Verify the Conversion

Verify the conversion by plugging in values or using algebraic manipulation to check the correctness of the converted equation.

Practical Examples of Equation Conversions

Here are some practical examples of equation conversions:

• Converting a Linear Equation to a Quadratic Equation: Convert the linear equation 2x + 3 = 5 to a quadratic equation.
• Converting a Quadratic Equation to a Linear Equation: Convert the quadratic equation x^2 + 4x + 4 = 0 to a linear equation.
• Converting a Polynomial Equation to a Rational Equation: Convert the polynomial equation x^3 + 2x^2 - 3x - 1 = 0 to a rational equation.

Benefits of Converting Equations

Converting equations has several benefits, including:

• Simplifying Complex Equations: Converting equations can simplify complex equations and reveal underlying patterns.
• Facilitating Problem-Solving: Converting equations can facilitate the solution of problems by providing a more manageable form.
• Revealing Properties and Behavior: Converting equations can reveal the properties and behavior of the system being modeled.

Common Mistakes to Avoid When Converting Equations

When converting equations, it's essential to avoid common mistakes, such as:

• Incorrect Application of Conversion Formula: Make sure to apply the correct conversion formula or technique.
• Insufficient Simplification: Ensure that the resulting equation is fully simplified.
• Failure to Verify the Conversion: Verify the conversion by plugging in values or using algebraic manipulation.

Conclusion

Converting equations is an essential skill in mathematics, physics, engineering, and other fields. By following the 5 easy steps outlined in this article, you can simplify the process and achieve the desired conversion. Remember to identify the type of conversion, write down the initial equation, apply the conversion formula, simplify the equation, and verify the conversion. By avoiding common mistakes and practicing regularly, you can become proficient in converting equations and tackle complex problems with confidence.