Convert Equations To Slope Intercept Form Easily

Quick Links :

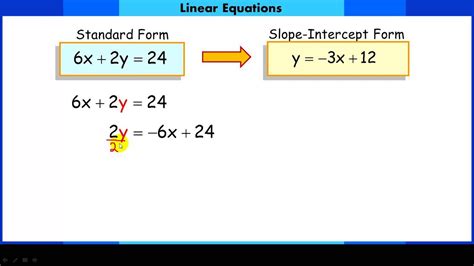
Converting equations to slope-intercept form is a fundamental skill in algebra and mathematics. It allows us to represent linear equations in a clear and concise manner, making it easier to analyze and solve problems. In this article, we will explore the importance of slope-intercept form, its benefits, and provide a step-by-step guide on how to convert equations to this form.

Understanding Slope-Intercept Form

Slope-intercept form is a way of expressing linear equations in the form y = mx + b, where m is the slope of the line and b is the y-intercept. This form is useful because it allows us to easily identify the slope and y-intercept of a line, which are essential in solving problems involving linear equations.

Benefits of Slope-Intercept Form

• Easily identify the slope and y-intercept of a line
• Simplifies the process of solving linear equations
• Allows for easy graphing of linear equations
• Facilitates the identification of parallel and perpendicular lines

Converting Equations to Slope-Intercept Form

Converting equations to slope-intercept form involves a few simple steps. Here's a step-by-step guide:

Step 1: Identify the Coefficients of x and y

Identify the coefficients of x and y in the given equation. The coefficient of x is the number that multiplies x, while the coefficient of y is the number that multiplies y.

Step 2: Rearrange the Equation

Rearrange the equation to isolate y on one side of the equation. This can be done by adding or subtracting the same value to both sides of the equation.

Step 3: Simplify the Equation

Simplify the equation by combining like terms. This will help to eliminate any unnecessary terms and make it easier to identify the slope and y-intercept.

Step 4: Write the Equation in Slope-Intercept Form

Write the equation in slope-intercept form by identifying the slope (m) and y-intercept (b). The slope is the coefficient of x, while the y-intercept is the constant term.

Example: Converting an Equation to Slope-Intercept Form

Consider the equation 2x + 3y = 7. To convert this equation to slope-intercept form, follow the steps outlined above.

Step 1: Identify the Coefficients of x and y

The coefficient of x is 2, while the coefficient of y is 3.

Step 2: Rearrange the Equation

Rearrange the equation to isolate y on one side of the equation. Subtract 2x from both sides of the equation to get:

3y = -2x + 7

Step 3: Simplify the Equation

Simplify the equation by dividing both sides by 3. This gives:

y = (-2/3)x + 7/3

Step 4: Write the Equation in Slope-Intercept Form

Write the equation in slope-intercept form by identifying the slope (m) and y-intercept (b). The slope is -2/3, while the y-intercept is 7/3.

The final equation in slope-intercept form is:

y = (-2/3)x + 7/3

Practical Applications of Slope-Intercept Form

Slope-intercept form has numerous practical applications in various fields, including physics, engineering, economics, and computer science. Here are a few examples:

• Physics: Slope-intercept form is used to describe the motion of objects in terms of their position, velocity, and acceleration.
• Engineering: Slope-intercept form is used to design and analyze systems, such as electrical circuits and mechanical systems.
• Economics: Slope-intercept form is used to model the behavior of economic systems, such as supply and demand curves.
• Computer Science: Slope-intercept form is used in computer graphics and game development to create 3D models and simulate real-world environments.

Common Mistakes to Avoid

When converting equations to slope-intercept form, there are a few common mistakes to avoid:

• Forgetting to simplify the equation
• Failing to identify the slope and y-intercept correctly
• Not rearranging the equation to isolate y

By avoiding these common mistakes, you can ensure that you convert equations to slope-intercept form accurately and efficiently.

Conclusion

Converting equations to slope-intercept form is a fundamental skill in algebra and mathematics. By following the steps outlined in this article, you can easily convert equations to slope-intercept form and take advantage of its numerous benefits. Remember to simplify the equation, identify the slope and y-intercept correctly, and avoid common mistakes.

We hope this article has been helpful in explaining the importance of slope-intercept form and providing a step-by-step guide on how to convert equations to this form. If you have any questions or need further clarification, please don't hesitate to ask.