5 Steps To Simplest Radical Form

Quick Links :

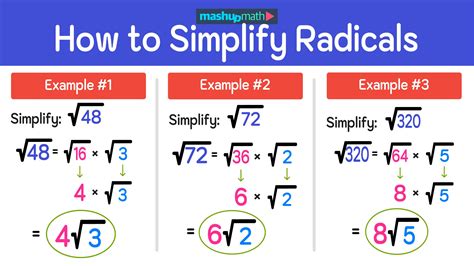
Radical expressions are an essential part of algebra and geometry, representing a way to express a number that cannot be simplified into a rational number. However, radical expressions can be quite complex, making them difficult to work with. To make them more manageable, we can simplify them into their simplest radical form. In this article, we will explore the 5 steps to simplest radical form.

Understanding Radical Expressions

Before we dive into the 5 steps to simplest radical form, it's essential to understand what radical expressions are and why we need to simplify them. Radical expressions represent a number that cannot be expressed as a rational number, such as the square root of a number that is not a perfect square.

For example, the square root of 2 is a radical expression because it cannot be simplified into a rational number. Radical expressions can be quite complex, making them difficult to work with in mathematical equations. Simplifying them into their simplest radical form makes it easier to perform mathematical operations.

What is Simplest Radical Form?

Simplest radical form is a way to express a radical expression in its most simplified form. This means that the radical expression is written in a way that has no perfect squares as factors of the radicand (the number inside the square root).

For example, the square root of 16 can be simplified into 4 because 16 is a perfect square. However, the square root of 2 cannot be simplified further because 2 is not a perfect square.

Step 1: Factor the Radicand

The first step to simplest radical form is to factor the radicand (the number inside the square root). Factoring the radicand means breaking it down into its prime factors.

For example, if we have the radical expression √24, we need to factor the radicand 24. The prime factors of 24 are 2 x 2 x 2 x 3. We can rewrite the radical expression as √(2 x 2 x 2 x 3).

Step 2: Identify Perfect Squares

The second step is to identify any perfect squares as factors of the radicand. Perfect squares are numbers that can be expressed as the square of an integer, such as 4 (2^2) or 9 (3^2).

Using our previous example, √(2 x 2 x 2 x 3), we can see that 2 x 2 is a perfect square because it can be expressed as 2^2. We can rewrite the radical expression as √(2^2 x 2 x 3).

Step 3: Simplify the Radical Expression

The third step is to simplify the radical expression by taking the square root of the perfect square. In our example, we can take the square root of 2^2, which gives us 2. We can rewrite the radical expression as 2√(2 x 3).

Step 4: Simplify the Radicand Further

The fourth step is to simplify the radicand further by factoring out any remaining perfect squares. In our example, we can see that 2 x 3 has no perfect squares as factors, so we cannot simplify the radicand further.

Step 5: Write the Final Answer

The final step is to write the final answer in simplest radical form. In our example, the final answer is 2√6.

Example Problems

Here are a few example problems to help you practice simplifying radical expressions into their simplest radical form:

• √48 =?
• √75 =?
• √200 =?

We hope this article has helped you understand the 5 steps to simplest radical form. By following these steps, you can simplify complex radical expressions and make them easier to work with in mathematical equations.