Radicals can be a daunting topic in mathematics, especially when it comes to simplifying them. However, with the right approach, simplifying radicals can be a breeze. In this article, we will break down the process of simplifying radicals into 5 easy steps, making it easier for you to understand and tackle radical expressions.
Radicals are an essential part of mathematics, and being able to simplify them is crucial for solving equations, functions, and other mathematical problems. Simplifying radicals involves expressing the radical in its simplest form, which can help to reduce errors and make calculations more manageable. Whether you're a student or a professional, mastering the art of simplifying radicals can make a significant difference in your mathematical endeavors.
Understanding Radicals

Before we dive into the 5 easy steps to simplify radicals, let's take a moment to understand what radicals are. A radical is a mathematical expression that contains a root, such as a square root, cube root, or nth root. Radicals are often used to express numbers that cannot be expressed as simple fractions. For example, the square root of 2 is a radical expression that represents a number that, when multiplied by itself, gives 2.
Step 1: Identify the Type of Radical

The first step in simplifying radicals is to identify the type of radical you're dealing with. There are two main types of radicals: square roots and nth roots. Square roots are the most common type of radical and are represented by the symbol √. Nth roots, on the other hand, are represented by the symbol √[n], where n is the index of the root. Identifying the type of radical will help you determine the correct approach to simplifying it.
Example:
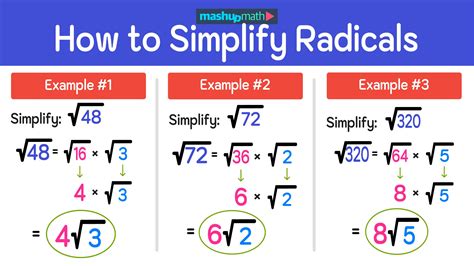
Identify the type of radical in the expression √16. Answer: The expression √16 is a square root radical.Step 2: Find the Largest Perfect Square or Cube

The next step in simplifying radicals is to find the largest perfect square or cube that divides the radicand (the number inside the radical). This is known as factoring out the perfect square or cube. For square roots, look for the largest perfect square that divides the radicand. For nth roots, look for the largest perfect nth power that divides the radicand.
Example:
Find the largest perfect square that divides the radicand in the expression √48. Answer: The largest perfect square that divides 48 is 16.Step 3: Write the Radical in Factored Form

Once you've identified the largest perfect square or cube that divides the radicand, write the radical in factored form. This involves expressing the radicand as a product of the perfect square or cube and a remaining factor.
Example:
Write the radical in factored form for the expression √48. Answer: √48 = √(16 × 3) = 4√3.Step 4: Simplify the Radical

The fourth step in simplifying radicals is to simplify the radical by combining any like terms and removing any remaining radicals.
Example:
Simplify the radical in the expression 4√3. Answer: The radical is already simplified.Step 5: Check Your Answer

The final step in simplifying radicals is to check your answer. Plug your simplified radical back into the original equation to ensure that it satisfies the equation.
Example:
Check the answer for the expression √48 = 4√3. Answer: Yes, the expression √48 = 4√3 satisfies the equation.By following these 5 easy steps, you can simplify radicals with ease. Remember to identify the type of radical, find the largest perfect square or cube, write the radical in factored form, simplify the radical, and check your answer.
We hope this article has helped you understand the process of simplifying radicals. If you have any questions or need further clarification, please don't hesitate to comment below.
What is a radical?
+A radical is a mathematical expression that contains a root, such as a square root, cube root, or nth root.
How do I simplify radicals?
+To simplify radicals, follow the 5 easy steps outlined in this article: identify the type of radical, find the largest perfect square or cube, write the radical in factored form, simplify the radical, and check your answer.
What is the difference between a square root and an nth root?
+A square root is a type of radical that represents a number that, when multiplied by itself, gives a specified value. An nth root, on the other hand, represents a number that, when raised to the power of n, gives a specified value.