5 Steps To Convert Vertex Form To Standard Form

Quick Links :

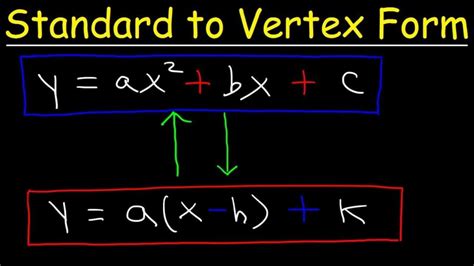
Understanding the Importance of Converting Vertex Form to Standard Form

In mathematics, quadratic equations are often represented in different forms, each with its own advantages and disadvantages. Two of the most commonly used forms are vertex form and standard form. The vertex form is useful for graphing and identifying the vertex of a parabola, while the standard form is more versatile and widely used in various mathematical operations. In this article, we will explore the 5 steps to convert vertex form to standard form, making it easier to work with quadratic equations in different contexts.

The process of converting vertex form to standard form is crucial in algebra and other branches of mathematics. It allows us to express quadratic equations in a more familiar and convenient way, making it easier to perform operations such as solving, graphing, and factoring. By mastering this conversion process, students and professionals can gain a deeper understanding of quadratic equations and develop problem-solving skills.

The Benefits of Converting Vertex Form to Standard Form

Converting vertex form to standard form offers several benefits, including:

• Simplifying complex equations: Standard form is often more straightforward and easier to work with, making it an ideal choice for solving quadratic equations.
• Enhancing graphing capabilities: By converting vertex form to standard form, we can take advantage of the latter's versatility in graphing and visualizing quadratic equations.
• Facilitating factoring and solving: Standard form is more conducive to factoring and solving quadratic equations, making it a valuable tool in algebra and other mathematical disciplines.

Step 1: Identify the Vertex Form of the Quadratic Equation

The first step in converting vertex form to standard form is to identify the vertex form of the quadratic equation. The vertex form is represented as:

y = a(x - h)^2 + k

where (h, k) is the vertex of the parabola, and a is a coefficient that determines the direction and width of the parabola.

Example:

Suppose we have the quadratic equation:

y = 2(x - 3)^2 + 1

In this case, the vertex form is already given, with (h, k) = (3, 1) and a = 2.

Step 2: Expand the Squared Term

The next step is to expand the squared term in the vertex form. This involves multiplying the squared term by the coefficient a and then expanding the resulting expression.

Using the example from Step 1:

y = 2(x - 3)^2 + 1

Expanding the squared term, we get:

y = 2(x^2 - 6x + 9) + 1

Resulting Expression:

y = 2x^2 - 12x + 18 + 1

Combine like terms to simplify the expression:

y = 2x^2 - 12x + 19

Step 3: Simplify the Expression

In this step, we simplify the expression obtained from expanding the squared term. This may involve combining like terms, removing any parentheses, and rearranging the terms to obtain the standard form.

Using the example from Step 2:

y = 2x^2 - 12x + 19

The expression is already simplified, so no further action is required.

Step 4: Rearrange the Terms (If Necessary)

In some cases, the expression obtained from simplifying may not be in the standard form. To convert it to standard form, we need to rearrange the terms to obtain the desired format.

Using the example from Step 3:

y = 2x^2 - 12x + 19

The expression is already in standard form, so no rearrangement is necessary.

Step 5: Verify the Standard Form

The final step is to verify that the expression obtained is indeed in the standard form. This involves checking that the expression is in the format ax^2 + bx + c, where a, b, and c are constants.

Using the example from Step 4:

y = 2x^2 - 12x + 19

The expression is indeed in standard form, with a = 2, b = -12, and c = 19.

Conclusion and Final Thoughts

Converting vertex form to standard form is an essential skill in mathematics, particularly in algebra and graphing. By following the 5 steps outlined in this article, you can master this conversion process and become proficient in working with quadratic equations.

To reinforce your understanding, try converting different vertex forms to standard form using the steps provided. You can also explore other mathematical concepts, such as factoring and solving quadratic equations, to further develop your skills.

If you have any questions or need clarification on any of the steps, feel free to ask in the comments below. Share this article with your friends and classmates who may benefit from learning about converting vertex form to standard form.

FAQ Section