3 Ways To Convert Slope Intercept To Standard Form

Quick Links :

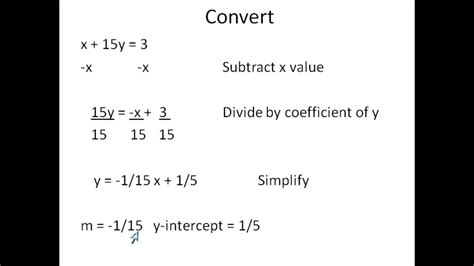
Converting slope-intercept form to standard form is an essential skill in algebra, and it can be accomplished in several ways. In this article, we will explore three methods to convert slope-intercept form to standard form, along with examples and explanations to help you master this skill.

Understanding Slope-Intercept Form and Standard Form

Before we dive into the methods, let's quickly review the slope-intercept form and standard form of a linear equation. The slope-intercept form is y = mx + b, where m is the slope and b is the y-intercept. The standard form, on the other hand, is Ax + By = C, where A, B, and C are integers.

Why Convert Slope-Intercept Form to Standard Form?

Converting slope-intercept form to standard form is necessary in various algebraic applications, such as solving systems of linear equations, graphing linear equations, and finding the equation of a line. Standard form is also useful when working with linear inequalities and quadratic equations.

Method 1: Rearranging Terms

One way to convert slope-intercept form to standard form is by rearranging the terms. This method involves moving the x term to the left side of the equation and the constant term to the right side.

Example: Convert y = 2x - 3 to standard form.

Rearrange the terms: 2x - y = 3

Add -2x to both sides: -2x + 2x - y = 3 + 2x

Simplify: -y = 3 + 2x

Multiply both sides by -1: y = -3 - 2x

Rearrange again: 2x + y = -3

This method is straightforward, but it requires careful attention to the signs and coefficients of the terms.

Method 2: Using the Slope and y-Intercept

Another method to convert slope-intercept form to standard form is by using the slope and y-intercept. This method involves finding the slope and y-intercept from the slope-intercept form and then using them to write the equation in standard form.

Example: Convert y = -3/2x + 2 to standard form.

Find the slope (m) and y-intercept (b): m = -3/2, b = 2

Write the equation in standard form: 3x + 2y = 4

This method is useful when you need to find the equation of a line given the slope and y-intercept.

Method 3: Using the x-Intercept

The third method involves finding the x-intercept of the line and using it to write the equation in standard form. The x-intercept is the point where the line crosses the x-axis, and it can be found by setting y = 0 in the slope-intercept form.

Example: Convert y = x - 2 to standard form.

Find the x-intercept: x - 2 = 0 --> x = 2

Write the equation in standard form: x - y = 2

This method is useful when you need to find the equation of a line given the x-intercept.

Conclusion

Converting slope-intercept form to standard form is an essential skill in algebra, and there are several methods to accomplish this. By using the methods outlined in this article, you can convert slope-intercept form to standard form with ease. Remember to practice regularly to become proficient in this skill.

Practical Applications

Converting slope-intercept form to standard form has many practical applications in real-life situations, such as:

• Finding the equation of a line given the slope and y-intercept
• Solving systems of linear equations
• Graphing linear equations
• Finding the x-intercept and y-intercept of a line

Final Thoughts

In conclusion, converting slope-intercept form to standard form is a valuable skill that can be achieved through practice and patience. By mastering this skill, you can tackle more complex algebraic problems and applications with confidence. So, keep practicing, and you'll become a pro at converting slope-intercept form to standard form in no time!