5 Ways To Master Fraction Slope Intercept Form

Quick Links :

Mastering the fraction slope-intercept form is a crucial milestone in algebra, enabling students to tackle complex equations with confidence. The slope-intercept form, y = mx + b, where m is the slope and b is the y-intercept, is a fundamental concept in linear equations. However, when fractions are introduced, the equation can become more intricate. In this article, we will delve into the world of fraction slope-intercept form and explore five ways to master this concept.

Understanding the Basics

Before we dive into the five ways to master fraction slope-intercept form, it's essential to understand the basics. The slope-intercept form represents a linear equation, where the slope (m) represents the rate of change, and the y-intercept (b) represents the point where the line intersects the y-axis. When fractions are introduced, the equation becomes more complex, requiring a deeper understanding of the underlying math.

**Method 1: Simplifying Fractions**

One of the most effective ways to master fraction slope-intercept form is to simplify fractions. This involves breaking down complex fractions into simpler ones, making it easier to work with the equation. For example, consider the equation:

y = (3/4)x + (2/5)

To simplify this equation, we can find the least common multiple (LCM) of the denominators, which is 20. Then, we can rewrite the equation as:

y = (15/20)x + (8/20)

Simplifying fractions is a crucial step in mastering fraction slope-intercept form, as it enables students to work with more manageable numbers.

**Benefits of Simplifying Fractions**

Simplifying fractions has several benefits, including:

• Easier to work with: Simplified fractions make it easier to perform arithmetic operations, such as addition and multiplication.
• Reduced errors: Simplifying fractions reduces the likelihood of errors, making it easier to solve equations accurately.
• Improved understanding: Simplifying fractions helps students understand the underlying math, making it easier to grasp more complex concepts.

**Method 2: Visualizing the Equation**

Another effective way to master fraction slope-intercept form is to visualize the equation. This involves graphing the equation on a coordinate plane, making it easier to understand the relationship between the variables. For example, consider the equation:

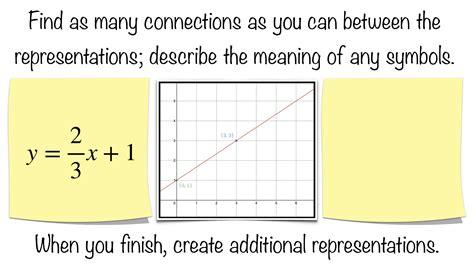
y = (2/3)x - (1/2)

To visualize this equation, we can graph it on a coordinate plane, using the slope (2/3) and y-intercept (-1/2) to plot the line. Visualizing the equation helps students understand the behavior of the line, making it easier to work with the equation.

**Benefits of Visualizing the Equation**

Visualizing the equation has several benefits, including:

• Improved understanding: Visualizing the equation helps students understand the relationship between the variables, making it easier to work with the equation.
• Easier to identify patterns: Visualizing the equation makes it easier to identify patterns, such as the slope and y-intercept.
• Reduced errors: Visualizing the equation reduces the likelihood of errors, making it easier to solve equations accurately.

**Method 3: Using Real-World Examples**

Using real-world examples is another effective way to master fraction slope-intercept form. This involves using everyday scenarios to illustrate the concept, making it more relatable and easier to understand. For example, consider a scenario where a car is traveling at a constant speed of 60 miles per hour, and the driver wants to know how far they will travel in 2 hours. This can be represented by the equation:

distance = (60/1)time

Using real-world examples helps students see the relevance of the concept, making it more engaging and easier to understand.

**Benefits of Using Real-World Examples**

Using real-world examples has several benefits, including:

• Improved engagement: Using real-world examples makes the concept more engaging and interesting, encouraging students to learn more.
• Easier to understand: Using real-world examples makes it easier for students to understand the concept, as it is more relatable to their everyday lives.
• Better retention: Using real-world examples helps students retain the information better, as it is more memorable.

**Method 4: Practicing with Different Types of Equations**

Practicing with different types of equations is another effective way to master fraction slope-intercept form. This involves working with various types of equations, such as linear, quadratic, and polynomial, to develop a deeper understanding of the concept. For example, consider the equation:

y = (2/3)x^2 - (1/2)x + 1

Practicing with different types of equations helps students develop a more comprehensive understanding of the concept, making it easier to work with more complex equations.

**Benefits of Practicing with Different Types of Equations**

Practicing with different types of equations has several benefits, including:

• Improved understanding: Practicing with different types of equations helps students develop a more comprehensive understanding of the concept.
• Easier to work with complex equations: Practicing with different types of equations makes it easier to work with more complex equations.
• Better problem-solving skills: Practicing with different types of equations helps students develop better problem-solving skills.

**Method 5: Using Online Resources**

Using online resources is another effective way to master fraction slope-intercept form. This involves using online tools, such as calculators and graphing software, to visualize and work with equations. For example, consider using a graphing calculator to visualize the equation:

y = (2/3)x - (1/2)

Using online resources helps students develop a more interactive and engaging learning experience, making it easier to understand and work with the concept.

**Benefits of Using Online Resources**

Using online resources has several benefits, including:

• Improved engagement: Using online resources makes the learning experience more engaging and interactive.
• Easier to understand: Using online resources helps students understand the concept better, as it provides a more visual and interactive learning experience.
• Better retention: Using online resources helps students retain the information better, as it is more memorable.

In conclusion, mastering fraction slope-intercept form requires a combination of simplifying fractions, visualizing the equation, using real-world examples, practicing with different types of equations, and using online resources. By using these methods, students can develop a deeper understanding of the concept, making it easier to work with more complex equations. We encourage you to share your thoughts and experiences with mastering fraction slope-intercept form in the comments section below.