5 Ways To Simplify Radicals

Quick Links :

Radicals, also known as roots, are a fundamental concept in mathematics, particularly in algebra and geometry. They represent a way to express a number that, when raised to a certain power, gives a specified value. However, working with radicals can sometimes be cumbersome, especially when dealing with complex expressions. In this article, we will explore five ways to simplify radicals, making it easier to work with these mathematical expressions.

Understanding Radicals

Before diving into the simplification methods, let's briefly review what radicals are and how they work. A radical is a mathematical symbol that represents the root of a number. The most common type of radical is the square root, which is denoted by the symbol √. For example, √16 represents the number that, when multiplied by itself, gives 16, which is 4.

Radicals can be expressed in different forms, including:

• Square root (√)
• Cube root (³√)
• Fourth root (⁴√)
• And so on

Method 1: Simplifying Square Roots

One of the most common methods of simplifying radicals is to look for perfect squares. A perfect square is a number that can be expressed as the square of an integer. For example, 16 is a perfect square because it can be expressed as 4².

To simplify a square root, look for the largest perfect square that divides the radicand (the number inside the radical). Then, take the square root of that perfect square and write it outside the radical.

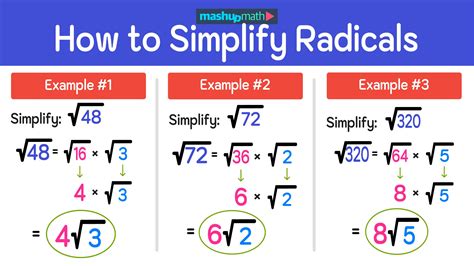
Example: Simplify √48

• Look for the largest perfect square that divides 48: 16
• Take the square root of 16: √16 = 4
• Write 4 outside the radical: √48 = 4√3

Working with Multiple Radicals

When working with multiple radicals, you can simplify them separately and then combine the results.

Example: Simplify √48 + √75

• Simplify √48: 4√3
• Simplify √75: 5√3
• Combine the results: 4√3 + 5√3 = 9√3

Method 2: Simplifying Cube Roots

Cube roots are similar to square roots, but they involve the cube of a number instead of the square. To simplify a cube root, look for perfect cubes. A perfect cube is a number that can be expressed as the cube of an integer.

Example: Simplify ³√64

• Look for the perfect cube that divides 64: 8³
• Take the cube root of 8³: ³√8³ = 2
• Write 2 outside the radical: ³√64 = 2³√2

Method 3: Simplifying Radicals with Coefficients

Radicals can also have coefficients, which are numbers that multiply the radical. To simplify a radical with a coefficient, you can factor the coefficient out of the radical.

Example: Simplify 2√48

• Factor the coefficient out of the radical: 2√48 = 2(√48)
• Simplify the radical: √48 = 4√3
• Write the simplified radical: 2(4√3) = 8√3

Method 4: Rationalizing the Denominator

When working with fractions that involve radicals, it's often necessary to rationalize the denominator. Rationalizing the denominator means getting rid of any radicals in the denominator.

Example: Simplify 1/√3

• Multiply the numerator and denominator by √3: 1/√3 × √3/√3 = √3/3
• Write the simplified fraction: √3/3

Method 5: Using Conjugates

Conjugates are pairs of binomials that have the same terms, but with opposite signs. Using conjugates can help simplify radicals by getting rid of any imaginary numbers.

Example: Simplify √(2 + √3)

• Find the conjugate of the binomial: 2 - √3
• Multiply the expression by the conjugate: √(2 + √3) × √(2 - √3) = √((2 + √3)(2 - √3))
• Simplify the expression: √(4 - 3) = √1 = 1

In conclusion, simplifying radicals can seem daunting, but with the right techniques, it can be made easier. By using these five methods, you can simplify radicals and make working with them more manageable. Remember to look for perfect squares, perfect cubes, and coefficients, and don't be afraid to rationalize the denominator or use conjugates when necessary.