5 Ways To Express Numbers In Simplest Radical Form

Quick Links :

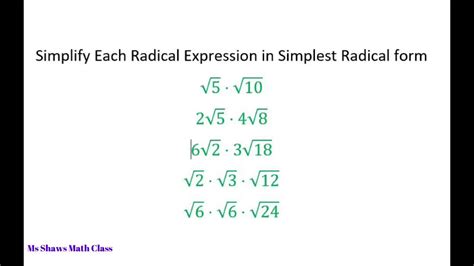
Expressing numbers in simplest radical form is an essential skill in mathematics, particularly in algebra and geometry. It involves simplifying radicals, which are expressions that contain square roots, cube roots, or other roots. Simplifying radicals is important because it allows us to work with numbers more easily and understand their properties better. In this article, we will explore five ways to express numbers in simplest radical form.

Understanding Radicals and Simplification

To begin with, let's define what radicals are. Radicals are mathematical expressions that contain roots, such as square roots, cube roots, or other types of roots. For example, √16 is a radical expression that represents the square root of 16. Simplifying radicals means finding the simplest form of the expression, which can be done by factoring out perfect squares or perfect cubes.

Benefits of Simplifying Radicals

Simplifying radicals has several benefits. It makes calculations easier, allows us to compare and order numbers more easily, and helps us understand the properties of numbers better. For example, simplifying radicals can help us determine whether a number is rational or irrational, and whether it can be expressed as a decimal or a fraction.

Five Ways to Express Numbers in Simplest Radical Form

Now, let's explore five ways to express numbers in simplest radical form.

1. Simplifying Square Roots

Simplifying square roots involves factoring out perfect squares from the radicand, which is the number inside the square root. For example, √16 can be simplified as √(4 × 4) = 4. Similarly, √24 can be simplified as √(4 × 6) = 2√6.

2. Simplifying Cube Roots

Simplifying cube roots involves factoring out perfect cubes from the radicand. For example, ³√27 can be simplified as ³√(3 × 3 × 3) = 3. Similarly, ³√64 can be simplified as ³√(4 × 4 × 4) = 4.

3. Using Conjugate Pairs

Conjugate pairs are pairs of numbers that have the same terms, but with opposite signs. Using conjugate pairs can help us simplify radicals by eliminating the radical sign. For example, √(a + b) can be simplified as (√a + √b)(√a - √b) / (√a - √b).

4. Rationalizing the Denominator

Rationalizing the denominator involves multiplying the numerator and denominator by a conjugate pair to eliminate the radical sign from the denominator. For example, 1 / √2 can be rationalized as (√2 / 2) × (√2 / √2) = √2 / 2.

5. Using Algebraic Manipulations

Algebraic manipulations involve using algebraic properties, such as the distributive property, to simplify radicals. For example, √(a + b) can be simplified as √a + √b using the distributive property.

Common Mistakes to Avoid

When simplifying radicals, there are several common mistakes to avoid. One common mistake is forgetting to simplify the radicand before simplifying the radical. Another mistake is simplifying the radical without factoring out perfect squares or perfect cubes.

Tips for Mastering Radical Simplification

To master radical simplification, practice is key. Here are some tips to help you improve your skills:

• Practice simplifying radicals with different types of numbers, such as perfect squares, perfect cubes, and irrational numbers.
• Use conjugate pairs and rationalizing the denominator to simplify radicals.
• Use algebraic manipulations to simplify radicals.
• Check your work carefully to avoid mistakes.

Conclusion - Take Your Math Skills to the Next Level

In conclusion, expressing numbers in simplest radical form is an important skill in mathematics. By mastering radical simplification, you can improve your math skills and take your problem-solving abilities to the next level. Remember to practice regularly, use conjugate pairs and rationalizing the denominator, and check your work carefully to avoid mistakes.

Call to Action - Share Your Thoughts and Questions

We hope this article has helped you understand the importance of expressing numbers in simplest radical form. Do you have any questions or thoughts on radical simplification? Share them with us in the comments below. We'd love to hear from you and help you improve your math skills.