Trigonometric form of complex numbers is a fundamental concept in mathematics, particularly in the field of algebra and trigonometry. It provides a powerful tool for simplifying complex expressions and solving equations involving complex numbers. In this article, we will explore the basics of complex numbers in trigonometric form, their benefits, and how to work with them.
What are Complex Numbers in Trigonometric Form?

Complex numbers in trigonometric form, also known as polar form, represent a complex number in terms of its magnitude (length) and angle (direction). This form is particularly useful when working with complex numbers that involve trigonometric functions, such as sine and cosine. A complex number in trigonometric form is written as:
z = r(cos θ + i sin θ)
where z is the complex number, r is the magnitude (length), θ is the angle (direction), and i is the imaginary unit.
Benefits of Trigonometric Form
The trigonometric form of complex numbers has several benefits, including:
- Simplifying complex expressions: Trigonometric form can be used to simplify complex expressions by converting them into a more manageable form.
- Solving equations: Trigonometric form can be used to solve equations involving complex numbers, particularly those that involve trigonometric functions.
- Visualizing complex numbers: Trigonometric form provides a visual representation of complex numbers, making it easier to understand their properties and behavior.
How to Convert Complex Numbers to Trigonometric Form

Converting a complex number to trigonometric form involves finding its magnitude and angle. Here are the steps:
- Find the magnitude (length): The magnitude of a complex number is given by the formula:
r = √(a^2 + b^2)
where a is the real part and b is the imaginary part of the complex number.
- Find the angle (direction): The angle of a complex number is given by the formula:
θ = arctan(b/a)
where a is the real part and b is the imaginary part of the complex number.
- Write the complex number in trigonometric form: Once you have found the magnitude and angle, you can write the complex number in trigonometric form as:
z = r(cos θ + i sin θ)
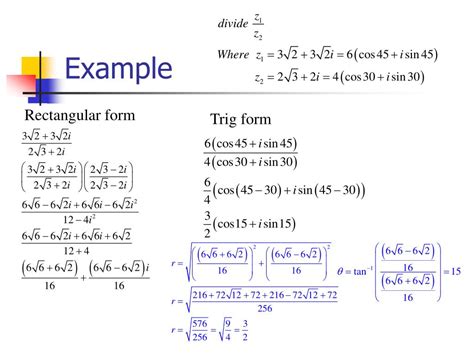
Example
Suppose we want to convert the complex number 3 + 4i to trigonometric form. We can follow the steps above:
- Find the magnitude:
r = √(3^2 + 4^2) = √(9 + 16) = √25 = 5
- Find the angle:
θ = arctan(4/3) = 53.13°
- Write the complex number in trigonometric form:
z = 5(cos 53.13° + i sin 53.13°)
Operations with Complex Numbers in Trigonometric Form

Complex numbers in trigonometric form can be added, subtracted, multiplied, and divided using the following rules:
- Addition and subtraction: Complex numbers in trigonometric form can be added and subtracted by converting them to rectangular form and performing the operation.
- Multiplication: Complex numbers in trigonometric form can be multiplied by multiplying their magnitudes and adding their angles.
- Division: Complex numbers in trigonometric form can be divided by dividing their magnitudes and subtracting their angles.
Example
Suppose we want to multiply the complex numbers 3(cos 30° + i sin 30°) and 2(cos 60° + i sin 60°). We can follow the rules above:
- Multiply the magnitudes: 3 × 2 = 6
- Add the angles: 30° + 60° = 90°
- Write the result in trigonometric form: 6(cos 90° + i sin 90°)
Applications of Complex Numbers in Trigonometric Form

Complex numbers in trigonometric form have numerous applications in mathematics, physics, and engineering. Some examples include:
- Signal processing: Trigonometric form is used in signal processing to represent and analyze signals.
- Control systems: Trigonometric form is used in control systems to represent and analyze systems.
- Electromagnetics: Trigonometric form is used in electromagnetics to represent and analyze electromagnetic waves.
Conclusion
Complex numbers in trigonometric form are a powerful tool for simplifying complex expressions and solving equations involving complex numbers. By understanding the benefits and how to work with complex numbers in trigonometric form, you can unlock a range of applications in mathematics, physics, and engineering. Whether you're a student or a professional, mastering complex numbers in trigonometric form can help you achieve your goals and succeed in your field.
What is the trigonometric form of a complex number?
+The trigonometric form of a complex number is a way of representing a complex number in terms of its magnitude (length) and angle (direction). It is written as z = r(cos θ + i sin θ), where z is the complex number, r is the magnitude, θ is the angle, and i is the imaginary unit.
How do I convert a complex number to trigonometric form?
+To convert a complex number to trigonometric form, you need to find its magnitude and angle. The magnitude is given by the formula r = √(a^2 + b^2), where a is the real part and b is the imaginary part of the complex number. The angle is given by the formula θ = arctan(b/a). Once you have found the magnitude and angle, you can write the complex number in trigonometric form as z = r(cos θ + i sin θ).
What are some applications of complex numbers in trigonometric form?
+Complex numbers in trigonometric form have numerous applications in mathematics, physics, and engineering. Some examples include signal processing, control systems, and electromagnetics.