5 Ways To Write Fractions In Expanded Form

Quick Links :

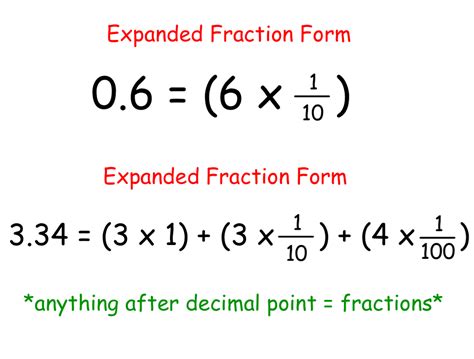
Expanded form is a mathematical concept that helps break down numbers into their place values, making it easier to understand and work with fractions. When it comes to writing fractions in expanded form, there are several ways to approach it. In this article, we will explore five different methods to write fractions in expanded form, providing you with a comprehensive understanding of this mathematical concept.

What is Expanded Form?

Before we dive into the different methods of writing fractions in expanded form, let's first understand what expanded form means. Expanded form is a way of expressing numbers as the sum of their place values. For example, the number 456 can be written in expanded form as 400 + 50 + 6. This helps us understand the value of each digit in the number.

Method 1: Decimal Expansion

One way to write fractions in expanded form is by using decimal expansion. This method involves converting the fraction into a decimal and then breaking it down into its place values. For example, the fraction 3/4 can be converted into a decimal as 0.75. Then, we can write it in expanded form as 0.7 + 0.05.

Steps to Follow:

• Convert the fraction into a decimal.
• Break down the decimal into its place values.
• Write the decimal in expanded form.

Method 2: Fractional Expansion

Another method to write fractions in expanded form is by using fractional expansion. This method involves breaking down the fraction into smaller fractions, making it easier to understand and work with. For example, the fraction 2/3 can be broken down into 1/3 + 1/3.

Steps to Follow:

• Break down the fraction into smaller fractions.
• Write the smaller fractions in expanded form.
• Combine the expanded forms to get the final result.

Method 3: Visual Representation

Visual representation is another effective way to write fractions in expanded form. This method involves using diagrams or pictures to represent the fraction, making it easier to understand and break down. For example, the fraction 1/2 can be represented as a circle divided into two equal parts.

Steps to Follow:

• Create a diagram or picture to represent the fraction.
• Break down the diagram into smaller parts.
• Write the fraction in expanded form based on the diagram.

Method 4: Numerical Expansion

Numerical expansion is a method that involves breaking down the fraction into its numerical values. For example, the fraction 3/4 can be broken down into 3 ÷ 4 = 0.75.

Steps to Follow:

• Divide the numerator by the denominator.
• Break down the result into its numerical values.
• Write the fraction in expanded form.

Method 5: Algebraic Expansion

Algebraic expansion is a method that involves using algebraic expressions to break down the fraction. For example, the fraction 2/3 can be expressed as 2 × 1/3.

Steps to Follow:

• Express the fraction as an algebraic expression.
• Break down the expression into smaller parts.
• Write the fraction in expanded form.

Writing fractions in expanded form can be done in various ways, and each method has its own advantages. By understanding and practicing these methods, you can develop a deeper understanding of fractions and improve your math skills.

Now that you have learned the different methods to write fractions in expanded form, try practicing with some examples. You can use the methods mentioned above to write fractions such as 1/2, 3/4, or 2/5 in expanded form.

If you have any questions or need further clarification on any of the methods, feel free to ask in the comments section below. Share this article with your friends and family who may be struggling with fractions, and help them develop a better understanding of this mathematical concept.