5 Ways To Master Expanded Exponential Form

Quick Links :

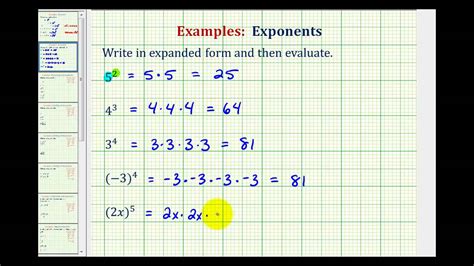
Expanded exponential form is a fundamental concept in mathematics, particularly in algebra and calculus. It involves expressing numbers in a way that simplifies complex calculations and provides a deeper understanding of mathematical relationships. Mastering expanded exponential form can be a game-changer for students and professionals alike, as it enables them to tackle challenging problems with ease and precision.

In this article, we will explore five ways to master expanded exponential form, from understanding the basics to applying advanced techniques. Whether you're a student looking to improve your math skills or a professional seeking to enhance your problem-solving abilities, this article will provide you with the knowledge and tools you need to succeed.

Understanding the Basics of Expanded Exponential Form

Expanded exponential form is a way of expressing numbers in the form of a x b, where a is the base and b is the exponent. For example, 2 x 3 x 4 can be expressed as 2^4 x 3. This form is particularly useful when dealing with large numbers or complex calculations, as it simplifies the process of multiplying and dividing.

To master expanded exponential form, it's essential to understand the basic rules and properties of exponents. These include:

• Product of powers: a^m x a^n = a^(m+n)
• Power of a power: (a^m)^n = a^(m x n)
• Power of a product: (a x b)^m = a^m x b^m

By understanding these properties, you can simplify complex expressions and perform calculations with ease.

Applying the Laws of Exponents

The laws of exponents are a set of rules that govern how exponents behave in different mathematical operations. These laws include:

• The product rule: a^m x a^n = a^(m+n)
• The quotient rule: a^m ÷ a^n = a^(m-n)
• The power rule: (a^m)^n = a^(m x n)

By applying these laws, you can simplify complex expressions and perform calculations with ease. For example:

• 2^3 x 2^4 = 2^(3+4) = 2^7
• 3^2 ÷ 3^1 = 3^(2-1) = 3^1
• (2^2)^3 = 2^(2 x 3) = 2^6

Using Expanded Exponential Form to Simplify Complex Expressions

Expanded exponential form is particularly useful when dealing with complex expressions involving multiple bases and exponents. By applying the laws of exponents, you can simplify these expressions and perform calculations with ease.

For example:

• 2^3 x 3^2 x 4^1 = 2^3 x 3^2 x 2^2 = 2^(3+2) x 3^2 = 2^5 x 3^2
• (2 x 3)^3 = 2^3 x 3^3
• 2^2 x 3^1 x 4^2 = 2^2 x 3^1 x 2^4 = 2^(2+4) x 3^1 = 2^6 x 3^1

By simplifying complex expressions using expanded exponential form, you can perform calculations with ease and precision.

Applying Expanded Exponential Form to Real-World Problems

Expanded exponential form has numerous applications in real-world problems, from finance to science. By mastering this concept, you can solve complex problems with ease and precision.

For example:

• Compound interest: A = P x (1 + r)^n, where A is the amount, P is the principal, r is the interest rate, and n is the number of years.
• Population growth: P = P0 x (1 + r)^n, where P is the population, P0 is the initial population, r is the growth rate, and n is the number of years.
• Chemical reactions: rate = k x [A]^m x [B]^n, where rate is the reaction rate, k is the rate constant, [A] and [B] are the concentrations of the reactants, and m and n are the orders of the reaction.

By applying expanded exponential form to these problems, you can simplify complex calculations and gain a deeper understanding of the underlying mathematical relationships.

Mastering Advanced Techniques in Expanded Exponential Form

Mastering advanced techniques in expanded exponential form requires a deep understanding of mathematical concepts and principles. Some advanced techniques include:

• Using logarithms to simplify complex expressions
• Applying the binomial theorem to expand complex expressions
• Using calculus to solve optimization problems

By mastering these advanced techniques, you can solve complex problems with ease and precision, and gain a deeper understanding of mathematical relationships.

Using Logarithms to Simplify Complex Expressions

Logarithms are a powerful tool for simplifying complex expressions involving exponents. By using logarithms, you can simplify complex expressions and perform calculations with ease.

For example:

• log(2^3 x 3^2) = log(2^3) + log(3^2) = 3log(2) + 2log(3)
• log((2 x 3)^4) = log(2^4 x 3^4) = 4log(2) + 4log(3)

By using logarithms to simplify complex expressions, you can perform calculations with ease and precision.

Conclusion and Final Thoughts

Mastering expanded exponential form is a fundamental skill that can benefit anyone who works with mathematics. By understanding the basics, applying the laws of exponents, simplifying complex expressions, applying advanced techniques, and using logarithms, you can solve complex problems with ease and precision.

We hope this article has provided you with a comprehensive guide to mastering expanded exponential form. Whether you're a student or a professional, we encourage you to practice and apply these concepts to real-world problems.

Leave a comment below and share your thoughts on how you use expanded exponential form in your work or studies.