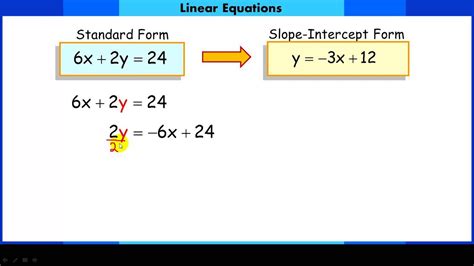
Converting Equations To Slope Intercept Form Easily

Quick Links :

Converting equations to slope-intercept form is a fundamental skill in algebra, and it's essential for solving linear equations, graphing lines, and understanding the relationships between variables. In this article, we'll explore the importance of slope-intercept form, its benefits, and provide a step-by-step guide on how to convert equations to slope-intercept form easily.

What is Slope-Intercept Form?

Slope-intercept form is a way of expressing linear equations in the form y = mx + b, where:

• m is the slope of the line, representing the rate of change between the x and y variables.
• b is the y-intercept, which is the point where the line intersects the y-axis.

Why is Slope-Intercept Form Important?

Slope-intercept form is essential in mathematics and real-life applications because it allows us to:

• Easily identify the slope and y-intercept of a line
• Graph lines quickly and accurately
• Solve linear equations and inequalities
• Model real-world situations, such as cost-benefit analysis, supply and demand, and physics problems

Benefits of Converting Equations to Slope-Intercept Form

Converting equations to slope-intercept form offers several benefits, including:

• Simplifies graphing and analysis of linear equations
• Enables easy identification of slope and y-intercept
• Facilitates solving linear equations and inequalities
• Enhances understanding of linear relationships and modeling

Step-by-Step Guide to Converting Equations to Slope-Intercept Form

To convert an equation to slope-intercept form, follow these steps:

1. Start with the given equation: Begin with the equation you want to convert, and identify the variables (x and y) and constants.
2. Add or subtract the same value to both sides: If the equation has a constant term on the same side as the variable term, add or subtract the same value to both sides to isolate the variable term.
3. Divide both sides by the coefficient of x: Divide both sides of the equation by the coefficient of x (the number multiplied by x) to solve for x.
4. Simplify the equation: Simplify the equation by combining like terms and removing any unnecessary parentheses or brackets.
5. Write the equation in slope-intercept form: The resulting equation should be in the form y = mx + b, where m is the slope and b is the y-intercept.

Examples of Converting Equations to Slope-Intercept Form

• Example 1: Convert the equation 2x + 3y = 7 to slope-intercept form.

Solution: Subtract 2x from both sides, then divide both sides by 3 to get y = (-2/3)x + 7/3.

• Example 2: Convert the equation x - 2y = -3 to slope-intercept form.

Solution: Add 2y to both sides, then divide both sides by 2 to get y = (1/2)x - 3/2.

Tips and Tricks for Converting Equations to Slope-Intercept Form

To make converting equations to slope-intercept form easier, keep the following tips in mind:

• Always start with the given equation and identify the variables and constants.
• Use inverse operations to isolate the variable term.
• Simplify the equation by combining like terms and removing unnecessary parentheses or brackets.
• Check your work by plugging in values for x and y to ensure the equation is true.

Common Mistakes to Avoid When Converting Equations to Slope-Intercept Form

• Forgetting to add or subtract the same value to both sides
• Dividing both sides by the wrong coefficient
• Failing to simplify the equation
• Not checking your work by plugging in values for x and y

Conclusion

Converting equations to slope-intercept form is a crucial skill in algebra and mathematics. By following the step-by-step guide and tips outlined in this article, you'll be able to easily convert equations to slope-intercept form and unlock the benefits of this powerful tool.

We hope this article has been informative and helpful in your journey to master slope-intercept form. If you have any questions or need further clarification, please don't hesitate to comment below. Share this article with your friends and classmates to help them understand the importance of slope-intercept form.