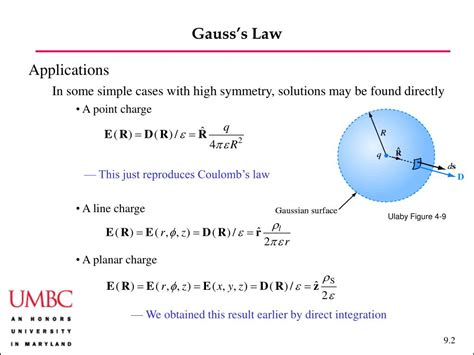
Gauss's Law is a fundamental concept in physics, particularly in the study of electromagnetism. It describes the relationship between electric charges and the resulting electric field. While Gauss's Law is often expressed in its integral form, it can also be expressed in differential form, which provides a more detailed understanding of the behavior of electric fields. In this article, we will delve into the differential form of Gauss's Law, exploring its derivation, significance, and applications.
Gauss's Law in Integral Form

Before diving into the differential form, let's briefly review Gauss's Law in its integral form. Gauss's Law states that the total electric flux through a closed surface is proportional to the charge enclosed within that surface. Mathematically, this is expressed as:
Φ = Q / ε₀
where Φ is the electric flux, Q is the charge enclosed, and ε₀ is the electric constant (permittivity of free space).
Derivation of Gauss's Law in Differential Form

To derive Gauss's Law in differential form, we start with the integral form and apply the divergence theorem. The divergence theorem states that the flux of a vector field through a closed surface is equal to the divergence of the field within the volume enclosed by the surface.
∇⋅E = ρ / ε₀
where E is the electric field, ρ is the charge density, and ε₀ is the electric constant.
Charge Density and Electric Field
The charge density ρ represents the amount of charge per unit volume. It can be expressed as:
ρ = Q / V
where Q is the charge and V is the volume.
The electric field E is a vector field that represents the force per unit charge at a given point in space. It can be expressed as:
E = F / q
where F is the force and q is the charge.
Significance of Gauss's Law in Differential Form

Gauss's Law in differential form provides a more detailed understanding of the behavior of electric fields. It allows us to:
- Determine the electric field at a point: By knowing the charge density and the electric constant, we can calculate the electric field at a given point in space.
- Study the behavior of electric fields: The differential form of Gauss's Law enables us to analyze the behavior of electric fields in various situations, such as the electric field around a point charge or the electric field within a conductor.
- Derive other fundamental laws: Gauss's Law in differential form can be used to derive other fundamental laws, such as the Lorentz force equation and the Poisson equation.
Applications of Gauss's Law in Differential Form
Gauss's Law in differential form has numerous applications in physics and engineering, including:
- Electromagnetic field theory: Gauss's Law is used to study the behavior of electromagnetic fields in various situations, such as the propagation of electromagnetic waves.
- Circuit analysis: Gauss's Law is used to analyze the behavior of electric circuits, including the calculation of voltage and current.
- Particle physics: Gauss's Law is used to study the behavior of subatomic particles, such as electrons and protons.
Conclusion: Unlocking the Secrets of Electric Fields

In conclusion, Gauss's Law in differential form provides a powerful tool for understanding the behavior of electric fields. By deriving the differential form from the integral form, we can gain a deeper understanding of the relationship between electric charges and the resulting electric field. The significance and applications of Gauss's Law in differential form make it an essential concept in physics and engineering.
We hope this article has provided you with a comprehensive understanding of Gauss's Law in differential form. If you have any questions or would like to share your thoughts, please comment below.
FAQ Section:
What is Gauss's Law in differential form?
+Gauss's Law in differential form is a mathematical expression that describes the relationship between electric charges and the resulting electric field. It states that the divergence of the electric field at a point is proportional to the charge density at that point.
What is the significance of Gauss's Law in differential form?
+Gauss's Law in differential form provides a more detailed understanding of the behavior of electric fields. It allows us to determine the electric field at a point, study the behavior of electric fields, and derive other fundamental laws.
What are the applications of Gauss's Law in differential form?
+Gauss's Law in differential form has numerous applications in physics and engineering, including electromagnetic field theory, circuit analysis, and particle physics.