3 Steps To Convert Slope Intercept To Standard Form

Quick Links :

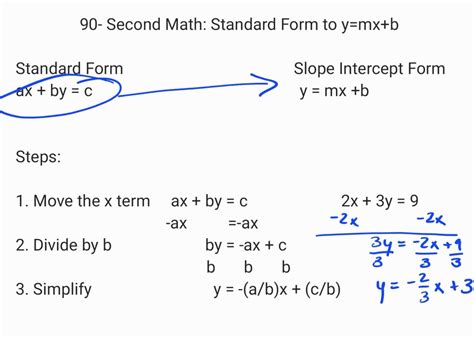
Converting linear equations from slope-intercept form to standard form is a fundamental concept in algebra and mathematics. Slope-intercept form, typically represented as y = mx + b, provides valuable information about a line's slope (m) and its y-intercept (b). However, standard form, often denoted as Ax + By = C, where A, B, and C are integers and A is non-negative, offers a comprehensive view of a line's characteristics. Understanding how to convert between these forms enhances problem-solving skills in various mathematical contexts.

Why Convert Between Forms?

Converting between slope-intercept and standard forms allows for a deeper understanding of linear equations. It's crucial for solving systems of linear equations, graphing lines, and interpreting the real-world implications of linear relationships. By being proficient in these conversions, individuals can approach problems from different angles, thereby expanding their analytical capabilities.

Step 1: Understanding the Forms

Understanding Slope-Intercept and Standard Forms

• Slope-Intercept Form (y = mx + b): This form clearly shows the slope (m) and the y-intercept (b) of a line. The slope indicates the rate of change, or how steep the line is, while the y-intercept is the point at which the line crosses the y-axis.

• Standard Form (Ax + By = C): In this form, A, B, and C are integers, and A is non-negative. This form is particularly useful for finding the x-intercept and for graphing lines using the intercept method.

Step 2: Converting Slope-Intercept to Standard Form

The Conversion Process

Converting from slope-intercept to standard form involves rearranging the equation so that the x and y terms are on one side, and the constant term is on the other side.

Here are the specific steps:

1. Move the x term to the left side of the equation: Start with the slope-intercept form y = mx + b. Subtract mx from both sides to get the x term by itself on one side: y - mx = b.

2. Move the y term to the left side and ensure integers: Rearrange to get y - mx + bx = b. This is essentially bringing all variables to one side and constants to the other, which aligns with the standard form structure.

3. Adjust coefficients to integers: If necessary, multiply the entire equation by a factor that will make all coefficients integers and ensure A (the coefficient of x) is non-negative.

Step 3: Practical Application

Example Conversion

Consider the equation y = 2x + 3 in slope-intercept form. To convert it into standard form:

1. Move the x term: Subtract 2x from both sides to get y - 2x = 3.

2. Adjust coefficients: Since 1 and -2 are already integers, and the coefficient of x is -2 (negative), we can multiply the entire equation by -1 to ensure the coefficient of x becomes positive: -y + 2x = -3.

3. Standard Form Adjustment: To fully comply with the standard form requirements (Ax + By = C), where A should be non-negative, we've achieved our goal: -y + 2x = -3.

This final equation, -y + 2x = -3, is in standard form. However, to strictly adhere to conventional representation (where the coefficient of x is positive), it's presented as is, understanding that standard form can vary slightly in its exact presentation rules.

Conclusion and Call to Action

Converting linear equations from slope-intercept to standard form is a straightforward yet powerful tool in algebra. By understanding and mastering this conversion, individuals can enhance their problem-solving skills and approach a wide range of mathematical and real-world problems with confidence.

Feel free to share your experiences with converting linear equations or ask questions about any challenges you face in the comments section below. Engaging with the mathematical community can further deepen your understanding and foster a supportive environment for learning.