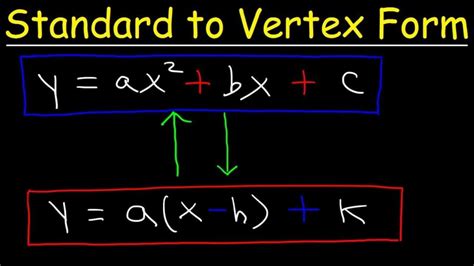
Converting the vertex form of a quadratic equation to the standard form is an essential skill in algebra. The vertex form of a quadratic equation is given by y = a(x - h)^2 + k, where (h, k) represents the vertex of the parabola. The standard form, on the other hand, is given by y = ax^2 + bx + c. In this article, we will explore three ways to convert the vertex form to the standard form.
Understanding the Vertex Form

Before we dive into the conversion methods, it's essential to understand the vertex form of a quadratic equation. The vertex form is given by y = a(x - h)^2 + k, where (h, k) represents the vertex of the parabola. The value of 'a' determines the direction and width of the parabola, while 'h' and 'k' determine the vertex's x and y coordinates, respectively.
Method 1: Expanding the Vertex Form

One way to convert the vertex form to the standard form is by expanding the squared term. This involves multiplying the binomial (x - h) by itself, which results in x^2 - 2hx + h^2. We can then distribute the coefficient 'a' to each term, resulting in ax^2 - 2ahx + ah^2. Finally, we add the constant term 'k' to obtain the standard form.
For example, consider the vertex form y = 2(x - 3)^2 + 1. Expanding the squared term, we get y = 2(x^2 - 6x + 9) + 1. Distributing the coefficient '2', we get y = 2x^2 - 12x + 18 + 1. Simplifying the equation, we get y = 2x^2 - 12x + 19, which is in the standard form.
Step-by-Step Process
- Expand the squared term (x - h)^2 to obtain x^2 - 2hx + h^2.
- Distribute the coefficient 'a' to each term, resulting in ax^2 - 2ahx + ah^2.
- Add the constant term 'k' to obtain the standard form.
Method 2: Using the Relationship Between Vertex and Standard Forms

Another way to convert the vertex form to the standard form is by using the relationship between the two forms. The standard form can be obtained by rearranging the vertex form. Specifically, we can rewrite the vertex form as y = a(x^2 - 2hx + h^2) + k. We can then simplify the equation by combining like terms.
For example, consider the vertex form y = 3(x - 2)^2 + 4. Rearranging the equation, we get y = 3(x^2 - 4x + 4) + 4. Expanding the squared term, we get y = 3x^2 - 12x + 12 + 4. Simplifying the equation, we get y = 3x^2 - 12x + 16, which is in the standard form.
Step-by-Step Process
- Rewrite the vertex form as y = a(x^2 - 2hx + h^2) + k.
- Simplify the equation by combining like terms.
- Rearrange the equation to obtain the standard form.
Method 3: Using a Graphing Calculator or Software

A third way to convert the vertex form to the standard form is by using a graphing calculator or software. Many graphing calculators and software programs, such as Desmos or Graphing Calculator, allow you to enter the vertex form of a quadratic equation and automatically convert it to the standard form.
For example, consider the vertex form y = 2(x - 3)^2 + 1. Using a graphing calculator or software, we can enter the equation and convert it to the standard form. The resulting equation will be y = 2x^2 - 12x + 19, which is in the standard form.
Step-by-Step Process
- Enter the vertex form of the quadratic equation into a graphing calculator or software.
- Use the calculator or software to convert the equation to the standard form.
- Verify the resulting equation.
What is the vertex form of a quadratic equation?
+The vertex form of a quadratic equation is given by y = a(x - h)^2 + k, where (h, k) represents the vertex of the parabola.
What is the standard form of a quadratic equation?
+The standard form of a quadratic equation is given by y = ax^2 + bx + c.
How do I convert the vertex form to the standard form?
+There are three ways to convert the vertex form to the standard form: expanding the vertex form, using the relationship between vertex and standard forms, and using a graphing calculator or software.
In conclusion, converting the vertex form of a quadratic equation to the standard form is a crucial skill in algebra. By using one of the three methods outlined in this article, you can easily convert the vertex form to the standard form. Whether you choose to expand the vertex form, use the relationship between vertex and standard forms, or use a graphing calculator or software, you will be able to convert the vertex form to the standard form with ease. So, take the first step and start practicing today!