Unlock Greens Theorem Circulation Form In 5 Easy Steps

Quick Links :

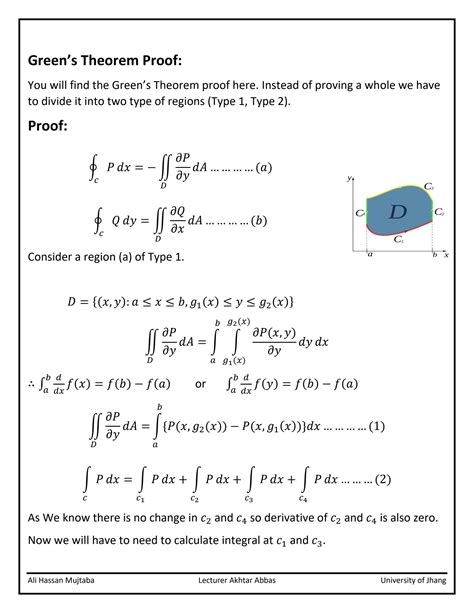
Green's Theorem is a fundamental concept in mathematics, particularly in the field of calculus. It is used to relate the line integral of a vector field around a closed curve to the double integral of the curl of the vector field over the region enclosed by the curve. In this article, we will explore the concept of Green's Theorem in circulation form and provide a step-by-step guide on how to unlock it.

Green's Theorem is a powerful tool that has numerous applications in physics, engineering, and other fields. It is used to calculate the circulation of a vector field around a closed curve, which is essential in understanding the behavior of electric and magnetic fields, fluid dynamics, and other physical phenomena. However, many students and professionals find it challenging to apply Green's Theorem in circulation form. In this article, we will break down the process into five easy steps, making it more accessible and easier to understand.

What is Green's Theorem?

Before we dive into the steps, let's briefly discuss what Green's Theorem is. Green's Theorem is a theorem in calculus that relates the line integral of a vector field around a closed curve to the double integral of the curl of the vector field over the region enclosed by the curve. It is named after the British mathematician George Green, who first introduced it in the 19th century.

Step 1: Understand the Circulation Form of Green's Theorem

The circulation form of Green's Theorem states that the line integral of a vector field around a closed curve is equal to the double integral of the curl of the vector field over the region enclosed by the curve. Mathematically, it can be expressed as:

∮C F · dr = ∬D (∂Q/∂x - ∂P/∂y) dA

where C is the closed curve, F is the vector field, D is the region enclosed by the curve, P and Q are the components of the vector field, and dA is the area element.

Step 2: Identify the Vector Field and the Closed Curve

To apply Green's Theorem, we need to identify the vector field and the closed curve. The vector field is a mathematical object that assigns a vector to each point in space. The closed curve is a curve that has no beginning or end and is used to enclose a region.

For example, let's consider a vector field F = (P, Q) = (x, -y) and a closed curve C that is a circle centered at the origin with radius 1.

Step 3: Calculate the Curl of the Vector Field

The curl of a vector field is a measure of how much the vector field rotates around a point. To calculate the curl, we need to find the partial derivatives of the components of the vector field.

For our example, the curl of the vector field F = (x, -y) is:

∂Q/∂x - ∂P/∂y = ∂(-y)/∂x - ∂x/∂y = 0 - 1 = -1

Step 4: Evaluate the Double Integral

Once we have calculated the curl of the vector field, we need to evaluate the double integral of the curl over the region enclosed by the curve.

For our example, the double integral is:

∬D (∂Q/∂x - ∂P/∂y) dA = ∬D (-1) dA = -∬D dA = -π(1)^2 = -π

Step 5: Find the Line Integral

Finally, we need to find the line integral of the vector field around the closed curve.

For our example, the line integral is:

∮C F · dr = ∮C (x, -y) · (dx, dy) = ∮C x dx - y dy

Using the parametric equation of the circle, we can evaluate the line integral:

∮C x dx - y dy = ∫[0,2π] cos(t) d(cos(t)) - sin(t) d(sin(t)) = -π

FAQ Section

In conclusion, Green's Theorem is a powerful tool that has numerous applications in physics, engineering, and other fields. By following the five easy steps outlined in this article, you can unlock the circulation form of Green's Theorem and apply it to a wide range of problems. Whether you are a student or a professional, mastering Green's Theorem will help you to better understand the behavior of electric and magnetic fields, fluid dynamics, and other physical phenomena.