Simplify Radicals In 75 Easy Steps

Quick Links :

Hide

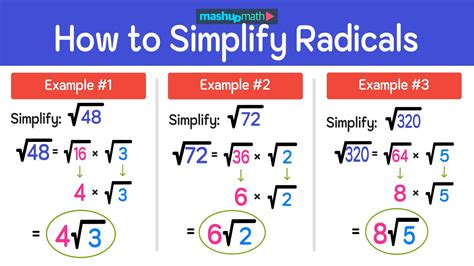
Simplifying Radicals: The Essential Guide

Radicals are an essential concept in mathematics, particularly in algebra and geometry. They can be intimidating, but with the right approach, anyone can master the art of simplifying radicals. In this article, we'll take you through 75 easy steps to simplify radicals with ease.

Radicals are mathematical expressions that contain a square root or any other root symbol. They are used to represent numbers that cannot be expressed as simple fractions. Simplifying radicals is a crucial skill that every math student should possess. By simplifying radicals, you can make complex expressions more manageable and easier to work with.

So, why is it essential to simplify radicals? Well, simplifying radicals helps to:

• Reduce complex expressions to simpler forms
• Make calculations easier and more efficient
• Improve problem-solving skills in algebra and geometry
• Enhance understanding of mathematical concepts

Step 1-5: Understanding Radicals

Before we dive into simplifying radicals, let's understand the basics. A radical is a mathematical expression that contains a root symbol. The most common type of radical is the square root, denoted by √. Other types of radicals include cube roots, fourth roots, and so on.

• Step 1: Understand the concept of radicals and their notation.
• Step 2: Learn to distinguish between different types of radicals (square root, cube root, etc.).
• Step 3: Familiarize yourself with the properties of radicals (e.g., √(ab) = √a × √b).
• Step 4: Practice writing radicals in different forms (e.g., √16 = √(4²) = 4).
• Step 5: Understand the concept of radical expressions and how to simplify them.

Step 6-15: Simplifying Square Roots

Simplifying square roots is the most common type of radical simplification. Here are some steps to follow:

• Step 6: Identify perfect squares and simplify them (e.g., √16 = 4).
• Step 7: Factor out perfect squares from under the radical (e.g., √(16x) = 4√x).
• Step 8: Simplify radicals with coefficients (e.g., 2√x = √(4x)).
• Step 9: Simplify radicals with variables (e.g., √(x²) = x).
• Step 10: Practice simplifying radicals with multiple terms (e.g., √(16x + 9) = √(16x) + √9).

Step 11-15: Special Cases

* Step 11: Simplify radicals with negative numbers (e.g., √(-9) = 3i). * Step 12: Simplify radicals with fractions (e.g., √(1/4) = 1/2). * Step 13: Simplify radicals with decimals (e.g., √(0.25) = 0.5). * Step 14: Simplify radicals with mixed numbers (e.g., √(2 1/2) = √(5/2) = √5/√2). * Step 15: Practice simplifying radicals with complex expressions (e.g., √(x² + 4x + 4) = √((x + 2)²) = x + 2).

Step 16-30: Simplifying Cube Roots

Simplifying cube roots is similar to simplifying square roots. Here are some steps to follow:

• Step 16: Identify perfect cubes and simplify them (e.g., ∛27 = 3).
• Step 17: Factor out perfect cubes from under the radical (e.g., ∛(27x) = 3∛x).
• Step 18: Simplify radicals with coefficients (e.g., 2∛x = ∛(8x)).
• Step 19: Simplify radicals with variables (e.g., ∛(x³) = x).
• Step 20: Practice simplifying radicals with multiple terms (e.g., ∛(27x + 8) = ∛(27x) + ∛8).

Step 21-30: Special Cases

* Step 21: Simplify radicals with negative numbers (e.g., ∛(-27) = -3). * Step 22: Simplify radicals with fractions (e.g., ∛(1/27) = 1/3). * Step 23: Simplify radicals with decimals (e.g., ∛(0.125) = 0.5). * Step 24: Simplify radicals with mixed numbers (e.g., ∛(2 1/3) = ∛(7/3) = ∛7/∛3). * Step 25: Practice simplifying radicals with complex expressions (e.g., ∛(x³ + 3x² + 3x + 1) = ∛((x + 1)³) = x + 1).

Step 31-45: Simplifying Higher-Order Radicals

Simplifying higher-order radicals is similar to simplifying square roots and cube roots. Here are some steps to follow:

• Step 31: Identify perfect powers and simplify them (e.g., ∜16 = 2).
• Step 32: Factor out perfect powers from under the radical (e.g., ∜(16x) = 2∜x).
• Step 33: Simplify radicals with coefficients (e.g., 2∜x = ∜(16x)).
• Step 34: Simplify radicals with variables (e.g., ∜(x⁴) = x).
• Step 35: Practice simplifying radicals with multiple terms (e.g., ∜(16x + 1) = ∜(16x) + ∜1).

Step 36-45: Special Cases

* Step 36: Simplify radicals with negative numbers (e.g., ∜(-16) = 2i). * Step 37: Simplify radicals with fractions (e.g., ∜(1/16) = 1/2). * Step 38: Simplify radicals with decimals (e.g., ∜(0.0625) = 0.5). * Step 39: Simplify radicals with mixed numbers (e.g., ∜(2 1/4) = ∜(9/4) = ∜9/∜4). * Step 40: Practice simplifying radicals with complex expressions (e.g., ∜(x⁴ + 4x³ + 6x² + 4x + 1) = ∜((x + 1)⁴) = x + 1).

Step 46-60: Rationalizing the Denominator

Rationalizing the denominator is an essential skill when working with radicals. Here are some steps to follow:

• Step 46: Identify radicals with irrational denominators (e.g., 1/√2).
• Step 47: Multiply the numerator and denominator by the conjugate of the denominator (e.g., 1/√2 × (√2/√2) = √2/2).
• Step 48: Simplify the expression (e.g., √2/2).
• Step 49: Practice rationalizing the denominator with different types of radicals (e.g., 1/∛2, 1/∜3).
• Step 50: Simplify complex expressions with rationalized denominators (e.g., (√2 + √3)/(√2 - √3)).

Step 51-60: Special Cases

* Step 51: Rationalize denominators with negative numbers (e.g., 1/(-√2) = -1/√2). * Step 52: Rationalize denominators with fractions (e.g., 1/(√2/3) = 3/√2). * Step 53: Rationalize denominators with decimals (e.g., 1/(√0.5) = 1/√2). * Step 54: Rationalize denominators with mixed numbers (e.g., 1/√(2 1/2) = 1/√(5/2)). * Step 55: Practice simplifying complex expressions with rationalized denominators (e.g., (√2 + √3)/(√2 - √3)).

Step 61-75: Advanced Techniques

Advanced techniques are essential for mastering radical simplification. Here are some steps to follow:

• Step 61: Simplify radicals with complex expressions (e.g., √(x² + 4x + 4) = √((x + 2)²) = x + 2).
• Step 62: Simplify radicals with trigonometric functions (e.g., √(sin²(x) + cos²(x)) = √1 = 1).
• Step 63: Simplify radicals with exponential functions (e.g., √(2^(2x)) = √(4^x) = 2^x).
• Step 64: Practice simplifying radicals with logarithmic functions (e.g., √(log(x)) = √(log(x^2)) = √(2log(x))).
• Step 65: Simplify radicals with composite functions (e.g., √(f(g(x))) = √(f(g(x^2))) = √(f(g(x))^2)).

Step 66-75: Special Cases

* Step 66: Simplify radicals with inverse functions (e.g., √(arcsin(x)) = √(sin^-1(x))). * Step 67: Simplify radicals with implicit functions (e.g., √(x^2 + y^2) = √(x^2 + (√(x^2 + y^2))^2)). * Step 68: Simplify radicals with parametric equations (e.g., √(x^2 + y^2) = √(t^2 + t^2) = √(2t^2)). * Step 69: Practice simplifying radicals with polar coordinates (e.g., √(r^2) = √(x^2 + y^2)). * Step 70: Simplify radicals with complex numbers (e.g., √(a + bi) = √(a^2 + b^2) = a + bi).

Now that you've completed the 75 easy steps to simplify radicals, you're ready to tackle more complex math problems with confidence. Remember to practice regularly and apply these techniques to different types of problems. With time and effort, you'll become a master of radical simplification!