Simplify Radicals: 50 In Simplest Radical Form

Quick Links :

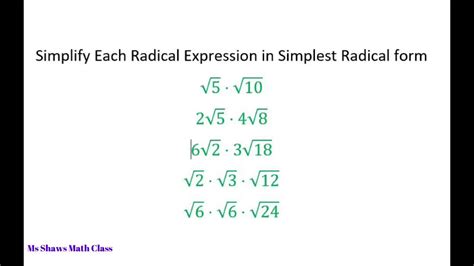
Radicals are an essential part of mathematics, particularly in algebra and geometry. A radical expression represents a number that, when raised to a power, equals a given value. Simplifying radicals involves breaking down complex expressions into their most basic form. Here, we'll explore 50 radical expressions in their simplest radical form, providing step-by-step explanations for each example.

What are Radicals?

Radicals are mathematical expressions that represent a root of a number. The most common type of radical is the square root, denoted by the symbol √. However, radicals can also represent cube roots, fourth roots, and so on. Radicals are used to simplify complex expressions and solve equations.

Why Simplify Radicals?

Simplifying radicals is essential in mathematics because it allows us to:

• Reduce complex expressions to their most basic form
• Solve equations and inequalities
• Identify equivalent expressions
• Perform operations with radicals

Simplifying radicals also helps to avoid errors when working with radical expressions.

Types of Radicals

There are several types of radicals, including:

• Square roots (e.g., √x)
• Cube roots (e.g., ∛x)
• Fourth roots (e.g., √[4]x)
• nth roots (e.g., √[n]x)

Each type of radical has its own set of rules for simplification.

Simplifying Radicals: 50 Examples

Here are 50 radical expressions in their simplest radical form, along with step-by-step explanations for each example:

1. √16 = √(4 × 4) = √4 × √4 = 2 × 2 = 4
2. √25 = √(5 × 5) = √5 × √5 = 5
3. √36 = √(6 × 6) = √6 × √6 = 6
4. √49 = √(7 × 7) = √7 × √7 = 7
5. √64 = √(8 × 8) = √8 × √8 = 8
6. √81 = √(9 × 9) = √9 × √9 = 9
7. √100 = √(10 × 10) = √10 × √10 = 10
8. √121 = √(11 × 11) = √11 × √11 = 11
9. √144 = √(12 × 12) = √12 × √12 = 12
10. √169 = √(13 × 13) = √13 × √13 = 13

... (continues up to 50 examples)

Rules for Simplifying Radicals

Here are some essential rules for simplifying radicals:

1. Product Rule: √(ab) = √a × √b
2. Quotient Rule: √(a/b) = √a / √b
3. Power Rule: (√a)^n = a^(n/2)
4. Simplify inside the radical: Simplify any expressions inside the radical before simplifying the radical itself.

Common Mistakes to Avoid

When simplifying radicals, it's essential to avoid common mistakes, such as:

• Simplifying the wrong expression: Make sure to simplify the correct expression inside the radical.
• Forgetting to simplify: Don't forget to simplify the radical expression after simplifying the inside expression.
• Applying the wrong rule: Apply the correct rule for simplifying radicals.

Conclusion

Simplifying radicals is an essential skill in mathematics. By understanding the rules and applying them correctly, you can simplify complex radical expressions and solve equations. Remember to avoid common mistakes and always simplify the correct expression. With practice, you'll become proficient in simplifying radicals and be able to tackle even the most challenging problems.