Breaking Down Complex Numbers into Simplest Form
Introduction to Simplifying Fractions

Simplifying fractions is a fundamental concept in mathematics that involves reducing a fraction to its simplest form. This process is essential in various mathematical operations, such as addition, subtraction, multiplication, and division of fractions. In this article, we will explore the steps involved in simplifying fractions and provide examples to illustrate the process.
What is a Fraction?

A fraction is a way to express a part of a whole as a ratio of two numbers. It consists of a numerator (the top number) and a denominator (the bottom number). For example, 3/4 is a fraction where 3 is the numerator and 4 is the denominator.
Types of Fractions
There are several types of fractions, including:
- Proper fractions: where the numerator is less than the denominator (e.g., 3/4)
- Improper fractions: where the numerator is greater than or equal to the denominator (e.g., 4/3)
- Mixed fractions: a combination of a whole number and a proper fraction (e.g., 2 3/4)
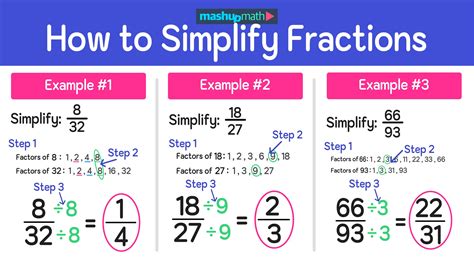
Steps to Simplify a Fraction

Simplifying a fraction involves dividing both the numerator and the denominator by the greatest common divisor (GCD). Here are the steps:
- Find the GCD: Determine the greatest common divisor of the numerator and the denominator.
- Divide both numbers: Divide both the numerator and the denominator by the GCD.
- Write the simplified fraction: Write the resulting fraction in simplest form.
Example 1: Simplifying a Proper Fraction
Simplify the fraction: 6/8
- Find the GCD: The GCD of 6 and 8 is 2.
- Divide both numbers: 6 ÷ 2 = 3 and 8 ÷ 2 = 4
- Write the simplified fraction: 3/4
Example 2: Simplifying an Improper Fraction
Simplify the fraction: 8/6
- Find the GCD: The GCD of 8 and 6 is 2.
- Divide both numbers: 8 ÷ 2 = 4 and 6 ÷ 2 = 3
- Write the simplified fraction: 4/3
Tips and Tricks for Simplifying Fractions

- Use prime factorization: Break down the numerator and denominator into their prime factors to find the GCD.
- Look for common factors: Identify any common factors between the numerator and denominator and cancel them out.
- Simplify before adding or subtracting: Simplify fractions before performing addition or subtraction operations.
Conclusion: Simplifying Fractions Made Easy

Simplifying fractions is a straightforward process that involves dividing both the numerator and the denominator by the greatest common divisor. By following the steps outlined in this article, you can simplify fractions with ease and confidence. Remember to use prime factorization, look for common factors, and simplify before performing addition or subtraction operations.
We hope this article has helped you understand the concept of simplifying fractions. Share your thoughts and feedback in the comments below!
What is the difference between a proper fraction and an improper fraction?
+A proper fraction has a numerator that is less than the denominator, while an improper fraction has a numerator that is greater than or equal to the denominator.
How do I find the greatest common divisor (GCD) of two numbers?
+The GCD can be found by listing the factors of each number and identifying the greatest common factor.
Can I simplify a fraction before adding or subtracting it from another fraction?
+Yes, it is recommended to simplify fractions before performing addition or subtraction operations to ensure accurate results.