43 As A Fraction: Simplified In 3 Easy Steps

Quick Links :

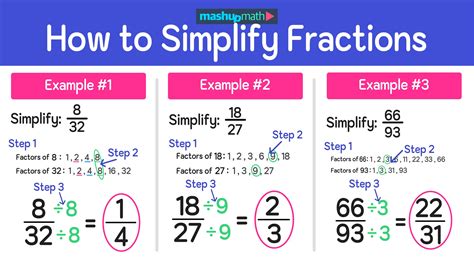
When we think about numbers, we often consider them as whole values, but sometimes we need to express them as fractions. A fraction is a way of representing a part of a whole as a ratio of two numbers. In this article, we will explore how to express 43 as a fraction in its simplest form.

Expressing numbers as fractions can seem daunting at first, but with the right approach, it can be a straightforward process. In this article, we will break down the steps to simplify 43 into a fraction, and by the end of it, you'll be a pro at converting numbers into their fractional forms.

From cooking recipes to mathematical equations, fractions are an essential part of our daily lives. Whether you're a student, a teacher, or just someone who loves numbers, understanding how to express numbers as fractions can open up a world of possibilities. So, let's dive in and explore how to simplify 43 into a fraction.

Step 1: Understanding the Basics of Fractions

Before we can simplify 43 into a fraction, we need to understand the basics of fractions. A fraction is a way of representing a part of a whole as a ratio of two numbers. The top number, known as the numerator, represents the part, while the bottom number, known as the denominator, represents the whole.

In the case of 43, we want to express it as a fraction in its simplest form. To do this, we need to find the greatest common divisor (GCD) of 43 and 1, since 43 is a whole number.

What is the Greatest Common Divisor (GCD)?

The GCD is the largest number that divides both numbers without leaving a remainder. In this case, the GCD of 43 and 1 is 1, since 1 is the only number that can divide both 43 and 1 without leaving a remainder.

Step 2: Finding the Fractional Form

Now that we have found the GCD, we can express 43 as a fraction. Since the GCD is 1, we can write 43 as 43/1. However, this is not the simplest form, as we can simplify it further.

To simplify the fraction, we need to find the smallest number that can divide both the numerator and the denominator. In this case, the smallest number that can divide both 43 and 1 is 1.

Therefore, the simplest form of 43 as a fraction is 43/1.

Why is 43/1 the Simplest Form?

The fraction 43/1 is the simplest form because it cannot be simplified further. Both the numerator and the denominator are divisible by 1, and there is no smaller number that can divide both.

Step 3: Verifying the Fraction

To verify that 43/1 is indeed the simplest form, we can check if it meets the following criteria:

• The numerator and denominator are integers.
• The denominator is non-zero.
• The fraction cannot be simplified further.

In this case, 43/1 meets all the criteria, and therefore, it is the simplest form of 43 as a fraction.

Real-World Applications of Fractions

Fractions have numerous real-world applications, from cooking recipes to mathematical equations. Understanding how to express numbers as fractions can help you in various aspects of your life, from measuring ingredients for a recipe to solving complex mathematical problems.

In conclusion, expressing 43 as a fraction in its simplest form requires understanding the basics of fractions, finding the GCD, and simplifying the fraction. By following these steps, you can simplify 43 into a fraction and unlock a world of possibilities.

We hope this article has helped you understand how to express 43 as a fraction. If you have any questions or comments, please feel free to share them below.