Simplify Fractions In 1 Easy Step

Quick Links :

Fractions can be a source of frustration for many students, but simplifying them doesn't have to be a daunting task. In fact, there's a simple trick that can help you simplify fractions in just one easy step. But before we dive into that, let's talk about why simplifying fractions is important and how it can make math problems easier to solve.

Why Simplify Fractions?

Simplifying fractions is an essential skill in mathematics because it helps to reduce complex fractions to their simplest form, making it easier to compare, add, subtract, multiply, and divide them. Simplifying fractions also helps to avoid confusion and errors when working with fractions in mathematical problems.

For example, let's say you have two fractions: 4/8 and 2/4. At first glance, these fractions may seem like different values, but when you simplify them, you'll find that they're actually equal. Simplifying fractions helps to reveal their true value, making it easier to work with them in mathematical problems.

The One Easy Step to Simplify Fractions

So, what's the one easy step to simplify fractions? It's quite simple, really. All you need to do is divide both the numerator and the denominator by their greatest common divisor (GCD). That's it!

Simplifying Fractions in 1 Easy Step

The greatest common divisor (GCD) is the largest number that divides both the numerator and the denominator without leaving a remainder. For example, if you have the fraction 6/8, the GCD of 6 and 8 is 2. So, to simplify the fraction, you would divide both the numerator and the denominator by 2, resulting in the simplified fraction 3/4.

How to Find the Greatest Common Divisor (GCD)

Finding the GCD is an essential part of simplifying fractions. There are a few ways to find the GCD, but here's a simple method:

1. List the factors of the numerator and the denominator.
2. Identify the common factors.
3. Choose the largest common factor, which is the GCD.

For example, let's say you have the fraction 12/18. To find the GCD, you would list the factors of 12 and 18:

Factors of 12: 1, 2, 3, 4, 6, 12 Factors of 18: 1, 2, 3, 6, 9, 18

The common factors are 1, 2, 3, and 6. The largest common factor is 6, which is the GCD.

Examples of Simplifying Fractions

Let's practice simplifying fractions using the one easy step method.

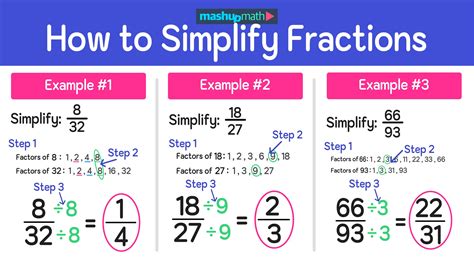
Example 1: Simplify the fraction 8/12.

GCD of 8 and 12: 4 Simplified fraction: 2/3

Example 2: Simplify the fraction 12/16.

GCD of 12 and 16: 4 Simplified fraction: 3/4

Example 3: Simplify the fraction 24/30.

GCD of 24 and 30: 6 Simplified fraction: 4/5

As you can see, simplifying fractions is a breeze when you know the one easy step method. Just remember to divide both the numerator and the denominator by their greatest common divisor, and you'll be simplifying fractions like a pro!

Benefits of Simplifying Fractions

Simplifying fractions has several benefits, including:

• Easier comparison: Simplified fractions make it easier to compare fractions and determine which one is larger or smaller.
• Easier calculation: Simplified fractions make calculations easier and less prone to errors.
• Better understanding: Simplifying fractions helps to reveal their true value, making it easier to understand mathematical concepts.

Real-World Applications of Simplifying Fractions

Simplifying fractions has real-world applications in various fields, including:

• Cooking: Simplifying fractions is essential in cooking, where recipes often involve fractional measurements.
• Science: Simplifying fractions is crucial in scientific calculations, where accuracy is paramount.
• Finance: Simplifying fractions is important in finance, where calculations involve interest rates, investments, and returns.

Conclusion

Simplifying fractions doesn't have to be a daunting task. With the one easy step method, you can simplify fractions in no time. Just remember to divide both the numerator and the denominator by their greatest common divisor, and you'll be on your way to becoming a fraction simplification pro!