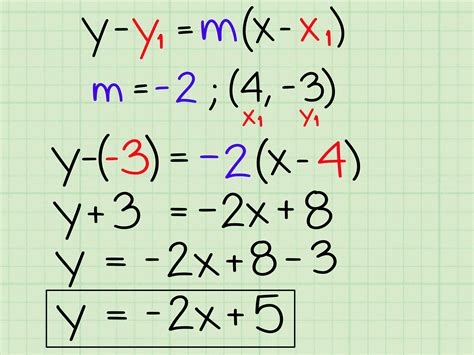Convert 3x Y 4 To Slope Intercept Form Easily

Quick Links :

Converting an equation from standard form to slope-intercept form can seem daunting, but with the right approach, it can be a breeze. In this article, we'll explore how to convert the equation 3x + 4y = 12 to slope-intercept form, and provide you with a step-by-step guide to make the process easier.

Why Convert to Slope-Intercept Form?

Before we dive into the conversion process, let's quickly discuss why slope-intercept form is useful. Slope-intercept form, also known as y = mx + b form, is a way of expressing linear equations that makes it easy to identify the slope (m) and y-intercept (b) of the line. This form is particularly useful for graphing lines, identifying the equation of a line given two points, and solving systems of linear equations.

Step 1: Understand the Standard Form

The given equation is 3x + 4y = 12, which is in standard form. In standard form, the equation is written as Ax + By = C, where A, B, and C are constants.

Step 2: Isolate the Variable y

To convert the equation to slope-intercept form, we need to isolate the variable y. We can do this by subtracting 3x from both sides of the equation, which gives us:

4y = -3x + 12

Step 3: Divide Both Sides by the Coefficient of y

Next, we need to divide both sides of the equation by the coefficient of y, which is 4. This gives us:

y = (-3/4)x + 3

Step 4: Identify the Slope and y-Intercept

Now that we have the equation in slope-intercept form, we can easily identify the slope (m) and y-intercept (b). In this case, the slope is -3/4, and the y-intercept is 3.

Practical Applications of Slope-Intercept Form

Slope-intercept form has numerous practical applications in real-life scenarios, such as:

• Graphing lines to visualize relationships between variables
• Identifying the equation of a line given two points
• Solving systems of linear equations
• Modeling real-world phenomena, such as population growth or financial transactions

Tips and Tricks for Converting to Slope-Intercept Form

Here are some tips and tricks to keep in mind when converting equations to slope-intercept form:

• Always isolate the variable y
• Divide both sides of the equation by the coefficient of y
• Identify the slope and y-intercept to gain a deeper understanding of the equation
• Use slope-intercept form to graph lines and solve systems of linear equations

Common Mistakes to Avoid

When converting equations to slope-intercept form, it's easy to make mistakes. Here are some common mistakes to avoid:

• Forgetting to isolate the variable y
• Failing to divide both sides of the equation by the coefficient of y
• Misidentifying the slope and y-intercept

Conclusion

Converting the equation 3x + 4y = 12 to slope-intercept form may seem challenging, but with the right approach, it's a breeze. By following the steps outlined in this article, you can easily convert equations to slope-intercept form and gain a deeper understanding of linear equations. Remember to always isolate the variable y, divide both sides of the equation by the coefficient of y, and identify the slope and y-intercept.

We hope this article has been informative and helpful. If you have any questions or comments, please don't hesitate to share them below.