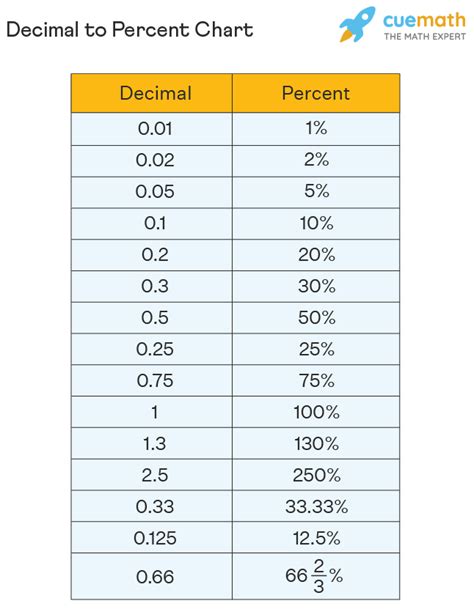
Converting percentages to decimals is a fundamental math operation that is used in various aspects of life, including finance, science, and everyday calculations. One of the most common conversions is 2 percent to decimal. In this article, we will explore the importance of converting percentages to decimals, the methods of conversion, and provide practical examples to help you master this skill.
Understanding Percentages and Decimals
Percentages and decimals are two different ways of representing numerical values. A percentage is a fraction of 100, represented by the symbol "%", while a decimal is a fraction of 1, represented by a point ".". Both are used to express proportions, but decimals are more commonly used in mathematical and scientific calculations.

The Importance of Converting Percentages to Decimals
Converting percentages to decimals is essential in various fields, including finance, science, and engineering. Decimals are more convenient for calculations, and they provide more precision. For example, in finance, decimal conversions are used to calculate interest rates, investment returns, and currency exchange rates. In science, decimals are used to express measurements, such as pH levels, temperatures, and concentrations.
Methods of Converting Percentages to Decimals
There are two common methods of converting percentages to decimals: the division method and the decimal place method.

Division Method
The division method involves dividing the percentage value by 100 to get the decimal equivalent.
Example: Convert 2% to decimal using the division method.
2 ÷ 100 = 0.02
Decimal Place Method
The decimal place method involves moving the decimal point two places to the left to get the decimal equivalent.
Example: Convert 2% to decimal using the decimal place method.
2% = 2.00 → 0.02
Practical Examples of 2 Percent in Decimal
Converting 2 percent to decimal is a common operation in various fields. Here are some practical examples:
- Finance: A savings account offers a 2% annual interest rate. If you deposit $1,000, how much interest will you earn in a year?
Interest = Principal x Rate = $1,000 x 0.02 = $20
- Science: A solution has a pH level of 2% acidity. If you need to express this value in decimal form, what is the equivalent value?
pH level = 2% = 0.02
- Everyday calculations: A store offers a 2% discount on all purchases. If you buy an item worth $100, how much will you pay after the discount?
Discount = Price x Rate = $100 x 0.02 = $2
Price after discount = $100 - $2 = $98
Benefits of Converting Percentages to Decimals
Converting percentages to decimals has several benefits, including:
- Simplifies calculations: Decimals are more convenient for calculations, especially when dealing with fractions and proportions.
- Provides precision: Decimals provide more precision than percentages, which is essential in scientific and mathematical calculations.
- Enhances understanding: Decimals help to enhance understanding of numerical values, making it easier to compare and analyze data.

Common Applications of 2 Percent in Decimal
Converting 2 percent to decimal has various applications in different fields, including:
- Finance: Interest rates, investment returns, and currency exchange rates.
- Science: Measurements, such as pH levels, temperatures, and concentrations.
- Everyday calculations: Discounts, taxes, and percentages.
Finance
In finance, 2 percent in decimal is used to calculate interest rates, investment returns, and currency exchange rates.
Example: A bank offers a 2% annual interest rate on a savings account. If you deposit $1,000, how much interest will you earn in a year?
Interest = Principal x Rate = $1,000 x 0.02 = $20
Science
In science, 2 percent in decimal is used to express measurements, such as pH levels, temperatures, and concentrations.
Example: A solution has a pH level of 2% acidity. If you need to express this value in decimal form, what is the equivalent value?
pH level = 2% = 0.02
Everyday calculations
In everyday calculations, 2 percent in decimal is used to calculate discounts, taxes, and percentages.
Example: A store offers a 2% discount on all purchases. If you buy an item worth $100, how much will you pay after the discount?
Discount = Price x Rate = $100 x 0.02 = $2
Price after discount = $100 - $2 = $98
Conclusion and Final Thoughts
Converting percentages to decimals is an essential math operation that is used in various aspects of life. Understanding how to convert 2 percent to decimal is crucial in finance, science, and everyday calculations. By mastering this skill, you can simplify calculations, provide precision, and enhance understanding of numerical values.

We hope this article has provided you with a comprehensive understanding of converting percentages to decimals, specifically 2 percent to decimal. If you have any questions or need further clarification, please feel free to comment below.
Now it's your turn! Share your thoughts and experiences with converting percentages to decimals. How do you use decimal conversions in your daily life?
What is the difference between a percentage and a decimal?
+A percentage is a fraction of 100, represented by the symbol "%", while a decimal is a fraction of 1, represented by a point ".". Both are used to express proportions, but decimals are more commonly used in mathematical and scientific calculations.
How do I convert a percentage to a decimal?
+There are two common methods of converting percentages to decimals: the division method and the decimal place method. The division method involves dividing the percentage value by 100, while the decimal place method involves moving the decimal point two places to the left.
What are the benefits of converting percentages to decimals?
+Converting percentages to decimals simplifies calculations, provides precision, and enhances understanding of numerical values. Decimals are more convenient for calculations, especially when dealing with fractions and proportions.