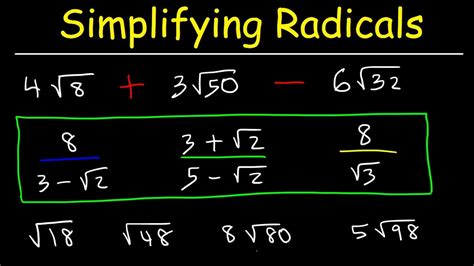
Simplifying radicals is a fundamental concept in mathematics, particularly in algebra and geometry. It involves breaking down complex radical expressions into simpler forms, making it easier to work with them in various mathematical operations. In this article, we will explore the three steps to simplify radicals and form more manageable expressions.
Understanding Radicals
Before diving into the steps to simplify radicals, it's essential to understand what radicals are. Radicals are mathematical expressions that contain a root, such as a square root, cube root, or nth root. The root is denoted by a symbol, such as √ or ∛, and it indicates the operation of finding a value that, when multiplied by itself, gives a specified number. For example, √16 = 4, because 4 multiplied by 4 equals 16.

Step 1: Factor Out Perfect Squares
The first step to simplify radicals is to factor out perfect squares from the expression inside the radical. A perfect square is a number that can be expressed as the product of an integer multiplied by itself. For example, 16 is a perfect square because it can be expressed as 4 × 4.
When simplifying radicals, look for perfect squares within the expression and factor them out. This will help reduce the complexity of the radical and make it easier to work with.
Example: Simplify √48
- Factor out perfect squares: 48 = 16 × 3
- Simplify: √48 = √(16 × 3) = √16 × √3 = 4√3
Step 2: Simplify the Radical Expression
Once you have factored out perfect squares, the next step is to simplify the radical expression. This involves reducing the radical to its simplest form by canceling out any common factors between the numerator and denominator.
When simplifying radical expressions, look for any common factors between the numerator and denominator and cancel them out. This will help reduce the complexity of the expression and make it easier to work with.
Example: Simplify √(24/8)
- Simplify the fraction: 24/8 = 3
- Simplify the radical: √3
Step 3: Rationalize the Denominator
The final step to simplify radicals is to rationalize the denominator. Rationalizing the denominator involves removing any radicals from the denominator by multiplying the expression by a clever form of 1.
When rationalizing the denominator, look for any radicals in the denominator and multiply the expression by a clever form of 1 that will eliminate the radical. This will help simplify the expression and make it easier to work with.
Example: Simplify √(3/√5)
- Multiply by a clever form of 1: √(3/√5) × (√5/√5) = √(3√5)/5

Benefits of Simplifying Radicals
Simplifying radicals has several benefits, including:
- Easier calculations: Simplifying radicals makes it easier to perform mathematical operations, such as addition, subtraction, multiplication, and division.
- Improved accuracy: Simplifying radicals reduces the likelihood of errors and improves accuracy in mathematical calculations.
- Enhanced understanding: Simplifying radicals helps to develop a deeper understanding of mathematical concepts and relationships.
Common Mistakes When Simplifying Radicals
When simplifying radicals, it's essential to avoid common mistakes, such as:
- Not factoring out perfect squares: Failing to factor out perfect squares can lead to more complex radical expressions.
- Not simplifying the radical expression: Failing to simplify the radical expression can lead to unnecessary complexity.
- Not rationalizing the denominator: Failing to rationalize the denominator can lead to radicals in the denominator, making it more challenging to work with the expression.

Conclusion
Simplifying radicals is an essential skill in mathematics, particularly in algebra and geometry. By following the three steps outlined in this article, you can simplify radical expressions and make them more manageable. Remember to factor out perfect squares, simplify the radical expression, and rationalize the denominator to simplify radicals effectively.
We hope this article has helped you understand the concept of simplifying radicals and how to apply it in various mathematical operations. If you have any questions or need further clarification, please don't hesitate to ask.
FAQ Section
What is the purpose of simplifying radicals?
+The purpose of simplifying radicals is to break down complex radical expressions into simpler forms, making it easier to work with them in various mathematical operations.
What is the first step to simplify radicals?
+The first step to simplify radicals is to factor out perfect squares from the expression inside the radical.
What is rationalizing the denominator?
+Rationalizing the denominator involves removing any radicals from the denominator by multiplying the expression by a clever form of 1.