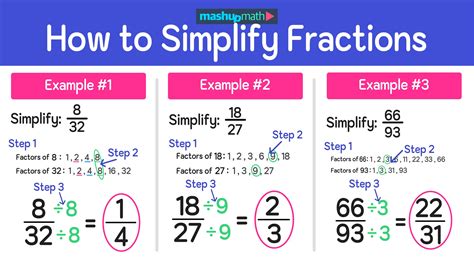
Simplifying 125 Over 1000: Fraction Reduction Made Easy

Quick Links :

Simplifying fractions is an essential math skill that can seem daunting, but with the right approach, it can be made easy. In this article, we will explore the concept of simplifying fractions, specifically 125 over 1000, and provide a step-by-step guide on how to reduce fractions to their simplest form.

Understanding Fractions

Before we dive into simplifying fractions, it's essential to understand the basics. A fraction is a way of expressing a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator tells us how many equal parts we have, and the denominator tells us how many parts the whole is divided into.

Why Simplify Fractions?

Simplifying fractions is crucial because it makes it easier to compare, add, subtract, multiply, and divide fractions. When fractions are in their simplest form, it's easier to understand the relationship between the numerator and the denominator. Simplifying fractions also helps to avoid errors and makes calculations more efficient.

Simplifying 125 over 1000

Now, let's take a look at the fraction 125 over 1000. To simplify this fraction, we need to find the greatest common divisor (GCD) of the numerator and the denominator.

Step 1: Find the Greatest Common Divisor (GCD)

To find the GCD of 125 and 1000, we can use the following methods:

• Listing the factors: List all the factors of 125 and 1000 and find the highest common factor.
• Using the Euclidean algorithm: This method involves dividing the larger number by the smaller number and finding the remainder.

Using the listing method, we can find that the factors of 125 are 1, 5, 25, and 125, and the factors of 1000 are 1, 2, 4, 5, 8, 10, 20, 25, 40, 50, 100, 125, 200, 250, 500, and 1000. The highest common factor is 125.

Step 2: Divide the Numerator and Denominator by the GCD

Now that we have found the GCD, we can simplify the fraction by dividing both the numerator and the denominator by 125.

Numerator: 125 ÷ 125 = 1 Denominator: 1000 ÷ 125 = 8

So, the simplified fraction is 1/8.

Benefits of Simplifying Fractions

Simplifying fractions has several benefits, including:

• Easier calculations: Simplifying fractions makes it easier to perform calculations, such as adding, subtracting, multiplying, and dividing.
• Improved understanding: Simplifying fractions helps to improve understanding of the relationship between the numerator and the denominator.
• Reduced errors: Simplifying fractions reduces the risk of errors and makes calculations more efficient.

Real-World Applications of Simplifying Fractions

Simplifying fractions has several real-world applications, including:

• Cooking: Simplifying fractions is essential in cooking, where recipes often involve fractions of ingredients.
• Science: Simplifying fractions is crucial in science, where calculations often involve fractions.
• Finance: Simplifying fractions is important in finance, where calculations often involve fractions of percentages.

Conclusion: Simplifying Fractions Made Easy

Simplifying fractions is an essential math skill that can seem daunting, but with the right approach, it can be made easy. By following the steps outlined in this article, you can simplify fractions with ease. Remember, simplifying fractions is crucial in various real-world applications, including cooking, science, and finance. With practice and patience, you can master the art of simplifying fractions.

Encourage Engagement:

We hope this article has helped you understand the concept of simplifying fractions and has provided you with a step-by-step guide on how to reduce fractions to their simplest form. If you have any questions or comments, please feel free to share them in the section below. Don't forget to share this article with your friends and family who may find it helpful.

FAQ Section: