Simplify Radicals: Expressing 100 In Simplest Radical Form

Quick Links :

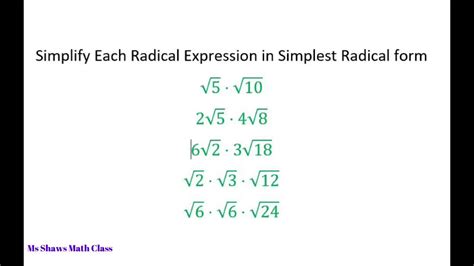
The concept of simplifying radicals is a fundamental aspect of mathematics, particularly in algebra and geometry. It involves expressing a radical expression in its simplest form, making it easier to work with and understand. In this article, we will delve into the world of simplifying radicals, focusing on expressing 100 in its simplest radical form.

What are Radicals?

Radicals, also known as roots, are mathematical expressions that represent a number raised to a fractional power. The most common type of radical is the square root, which is denoted by the symbol √. For example, √16 represents the number that, when multiplied by itself, equals 16. In this case, the square root of 16 is 4, because 4 × 4 = 16.

Why Simplify Radicals?

Simplifying radicals is essential in mathematics because it allows us to work with expressions more efficiently. When radicals are in their simplest form, it becomes easier to perform mathematical operations such as addition, subtraction, multiplication, and division. Moreover, simplifying radicals helps to avoid errors and confusion that can arise when working with complex expressions.

Simplifying Radicals: The Basics

To simplify a radical expression, we need to follow certain rules and guidelines. The first step is to look for any perfect squares or perfect cubes that can be factored out of the expression. For example, √16 can be simplified as √(4 × 4), which equals 4. This is because 4 is a perfect square, and we can take the square root of it.

The Perfect Square Rule

The perfect square rule states that if we have a radical expression of the form √(a × b), where a is a perfect square, we can simplify it as √a × √b. For example, √(16 × 2) can be simplified as √16 × √2, which equals 4√2.

Expressing 100 in Simplest Radical Form

Now, let's focus on expressing 100 in its simplest radical form. To do this, we need to find the largest perfect square that divides 100. In this case, the largest perfect square that divides 100 is 25, because 100 = 25 × 4.

Using the Perfect Square Rule

Using the perfect square rule, we can simplify √100 as √(25 × 4), which equals √25 × √4. Since √25 = 5 and √4 = 2, we can simplify further as 5 × 2 = 10.

Simplest Radical Form of 100

Therefore, the simplest radical form of 100 is 10. This means that √100 can be expressed as 10, making it easier to work with in mathematical expressions.

Key Takeaways

In summary, simplifying radicals is an essential aspect of mathematics that involves expressing a radical expression in its simplest form. By following the perfect square rule and factoring out perfect squares, we can simplify radicals and make mathematical operations more efficient. In the case of expressing 100 in its simplest radical form, we found that √100 = 10.

Practical Applications of Simplifying Radicals

Simplifying radicals has numerous practical applications in various fields, including physics, engineering, and computer science. In physics, simplifying radicals is used to calculate distances, velocities, and accelerations. In engineering, it is used to design and optimize systems, such as bridges and electronic circuits. In computer science, simplifying radicals is used in algorithms and data structures.

Real-World Examples

For example, in physics, the distance traveled by an object under constant acceleration can be calculated using the equation d = √(2as), where d is the distance, a is the acceleration, and s is the initial velocity. By simplifying the radical expression, we can make the calculation more efficient and accurate.

Common Mistakes to Avoid

When simplifying radicals, there are common mistakes to avoid. One of the most common mistakes is failing to factor out perfect squares. Another mistake is simplifying radicals incorrectly, which can lead to errors and confusion.

Best Practices

To avoid these mistakes, it is essential to follow best practices when simplifying radicals. First, always look for perfect squares and perfect cubes that can be factored out. Second, use the perfect square rule to simplify radicals. Finally, double-check your calculations to ensure accuracy.

Conclusion

In conclusion, simplifying radicals is a fundamental aspect of mathematics that involves expressing a radical expression in its simplest form. By following the perfect square rule and factoring out perfect squares, we can simplify radicals and make mathematical operations more efficient. Expressing 100 in its simplest radical form, we found that √100 = 10. Simplifying radicals has numerous practical applications in various fields, and by following best practices, we can avoid common mistakes and ensure accuracy.

Final Thoughts

We hope this article has provided you with a deeper understanding of simplifying radicals and expressing 100 in its simplest radical form. Remember to always follow best practices and double-check your calculations to ensure accuracy. If you have any questions or comments, please feel free to share them below.