0.6 As A Fraction Simplified

Quick Links :

The importance of understanding fractions cannot be overstated, especially when it comes to simplifying them. A fraction is a way of expressing a part of a whole, and simplifying fractions is a crucial math skill that can be applied in various real-life situations. In this article, we will delve into the world of fractions and explore the concept of 0.6 as a fraction simplified.

What is a Fraction?

A fraction is a way of expressing a part of a whole. It consists of two parts: the numerator and the denominator. The numerator represents the number of equal parts that we have, while the denominator represents the total number of parts that the whole is divided into. For example, the fraction 3/4 represents 3 equal parts out of a total of 4 parts.

Why Simplify Fractions?

Simplifying fractions is essential because it helps us to express the fraction in its most basic form. This makes it easier to compare fractions, add and subtract them, and perform other mathematical operations. Simplifying fractions also helps us to avoid confusion and errors when working with fractions.

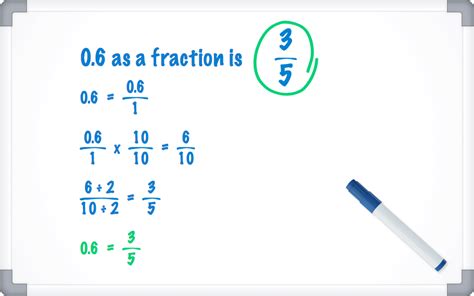
Converting 0.6 to a Fraction

To convert 0.6 to a fraction, we can use the following steps:

• Multiply 0.6 by 10 to get 6.
• Divide 6 by 10 to get 6/10.
• Simplify the fraction 6/10 by dividing both the numerator and the denominator by 2.

Using these steps, we can simplify 0.6 to 3/5.

Why is 3/5 the Simplified Form of 0.6?

The fraction 3/5 is the simplified form of 0.6 because it expresses the same value in its most basic form. When we simplify a fraction, we divide both the numerator and the denominator by the greatest common divisor (GCD). In this case, the GCD of 6 and 10 is 2, so we divide both numbers by 2 to get 3/5.

Real-World Applications of Simplifying Fractions

Simplifying fractions has numerous real-world applications. For example, in cooking, fractions are used to measure ingredients. If a recipe calls for 3/4 cup of flour, simplifying the fraction to 3/4 makes it easier to measure out the right amount. In finance, fractions are used to express interest rates and investment returns. Simplifying these fractions helps investors to make informed decisions.

Practical Examples of Simplifying Fractions

Here are some practical examples of simplifying fractions:

• 2/4 = 1/2 (simplifying a fraction by dividing both numbers by 2)
• 6/8 = 3/4 (simplifying a fraction by dividing both numbers by 2)
• 9/12 = 3/4 (simplifying a fraction by dividing both numbers by 3)

Benefits of Simplifying Fractions

Simplifying fractions has several benefits, including:

• Easier comparison of fractions
• Simplified addition and subtraction of fractions
• Improved accuracy when working with fractions
• Enhanced understanding of mathematical concepts

Common Mistakes to Avoid When Simplifying Fractions

When simplifying fractions, it's essential to avoid common mistakes, such as:

• Dividing both numbers by the wrong number
• Forgetting to simplify the fraction
• Not checking for equivalent fractions

Conclusion and Next Steps

In conclusion, simplifying fractions is a crucial math skill that can be applied in various real-life situations. By understanding the concept of fractions and how to simplify them, we can improve our math skills and make informed decisions. We encourage you to practice simplifying fractions and explore real-world applications of this concept.

Take the Next Step

Take the next step in improving your math skills by practicing simplifying fractions. Try simplifying different fractions and exploring real-world applications of this concept. Share your experiences and tips with others and continue to learn and grow.