3 Ways To Simplify 0.14 As A Fraction

Quick Links :

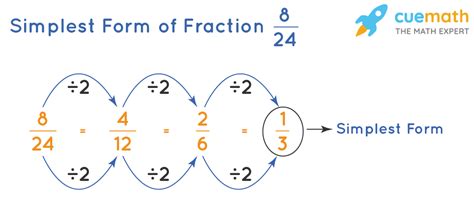
Simplifying fractions is an essential skill in mathematics, and it can be a challenging task, especially when dealing with decimals. The decimal 0.14 can be simplified as a fraction in three different ways.

Understanding the Basics of Fractions

Before diving into the methods, it's essential to understand what fractions represent. A fraction is a way to express a part of a whole as a ratio of two numbers. The top number, known as the numerator, represents the number of equal parts, while the bottom number, known as the denominator, represents the total number of parts.

Method 1: Converting Decimal to Fraction

The simplest way to simplify 0.14 as a fraction is to convert it directly. Since 0.14 is a decimal, we can express it as 14/100. To simplify this fraction, we need to find the greatest common divisor (GCD) of 14 and 100.

Calculating the Greatest Common Divisor (GCD)

To find the GCD of 14 and 100, we can use the Euclidean algorithm or simply list the factors of each number. The factors of 14 are 1, 2, 7, and 14, while the factors of 100 are 1, 2, 4, 5, 10, 20, 25, 50, and 100. The greatest common divisor is 2.

Simplifying the Fraction

Now that we have the GCD, we can simplify the fraction by dividing both the numerator and denominator by 2. This gives us:

14 ÷ 2 = 7 100 ÷ 2 = 50

So, 0.14 can be simplified as 7/50.

Method 2: Using Equivalent Ratios

Another way to simplify 0.14 as a fraction is by using equivalent ratios. Since 0.14 is equal to 14/100, we can multiply both the numerator and denominator by the same number to get an equivalent ratio.

For example, if we multiply both the numerator and denominator by 2, we get:

14 × 2 = 28 100 × 2 = 200

So, 0.14 is equal to 28/200. We can simplify this fraction further by dividing both numbers by 4, resulting in:

28 ÷ 4 = 7 200 ÷ 4 = 50

Again, we get the simplified fraction 7/50.

Method 3: Using Visual Models

The third method involves using visual models to simplify 0.14 as a fraction. Imagine a pizza with 100 equal slices, where 14 slices are shaded. To simplify this fraction, we can divide the pizza into smaller groups.

For example, we can divide the pizza into groups of 2 slices each. Since there are 50 groups, and 7 groups are shaded, we can express this as 7/50.

Real-World Applications

Simplifying fractions is not just a mathematical concept; it has real-world applications in various fields. For instance, in cooking, recipes often involve fractions of ingredients. Simplifying these fractions can help with measurements and scaling recipes.

Conclusion

In conclusion, simplifying 0.14 as a fraction can be done using three different methods: converting decimal to fraction, using equivalent ratios, and using visual models. Each method involves finding the greatest common divisor and simplifying the fraction to its lowest terms. Understanding fractions and how to simplify them is an essential skill in mathematics, with real-world applications in various fields.

We encourage you to try out these methods with different decimals and fractions to become more comfortable with simplifying fractions.

Share your thoughts: Which method do you find most helpful for simplifying fractions? Do you have any real-world examples of using fractions? Share your comments and questions below!