The Importance of Simplifying Ratios

Ratios are a fundamental concept in mathematics, and simplifying them is an essential skill to master. In this article, we will explore the process of simplifying ratios, with a specific focus on the ratio 50:10. We will delve into the reasons why simplifying ratios is crucial, the steps involved in the process, and provide practical examples to illustrate the concept.
Why Simplify Ratios?
Simplifying ratios is important for several reasons. Firstly, it helps to reduce complexity and make comparisons easier. When ratios are simplified, it becomes clearer how the different quantities relate to each other. This, in turn, facilitates decision-making and problem-solving. Secondly, simplifying ratios is essential in various fields, such as science, engineering, and finance, where accurate comparisons and calculations are critical.
The Process of Simplifying Ratios

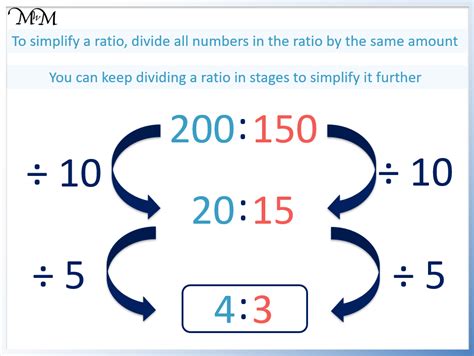
Simplifying ratios involves finding the greatest common divisor (GCD) of the two numbers and dividing both numbers by the GCD. The GCD is the largest number that divides both numbers without leaving a remainder.
Step-by-Step Guide to Simplifying the Ratio 50:10
- Identify the two numbers in the ratio: 50 and 10.
- Find the greatest common divisor (GCD) of 50 and 10. The factors of 50 are 1, 2, 5, 10, 25, and 50, while the factors of 10 are 1, 2, 5, and 10. The greatest common divisor is 10.
- Divide both numbers by the GCD: 50 ÷ 10 = 5 and 10 ÷ 10 = 1.
- Write the simplified ratio: 5:1.
Benefits of Simplifying the Ratio 50:10

Simplifying the ratio 50:10 to 5:1 has several benefits. Firstly, it makes the comparison easier to understand and interpret. Secondly, it facilitates calculations and problem-solving. For instance, if you need to calculate the proportion of 50 to 10 in a real-world scenario, using the simplified ratio 5:1 makes the calculation more manageable.
Real-World Applications of Simplifying Ratios

Simplifying ratios has numerous real-world applications. In finance, simplified ratios help investors and analysts evaluate the performance of companies and make informed decisions. In science, simplified ratios facilitate calculations and comparisons in various fields, such as physics, chemistry, and biology. In engineering, simplified ratios are essential for designing and optimizing systems, structures, and processes.
Common Mistakes to Avoid When Simplifying Ratios

When simplifying ratios, there are common mistakes to avoid. Firstly, ensure that you find the greatest common divisor (GCD) correctly. Secondly, make sure to divide both numbers by the GCD. Finally, double-check your calculations to avoid errors.
Conclusion
In conclusion, simplifying ratios is an essential skill that has numerous benefits and applications. By following the steps outlined in this article, you can simplify the ratio 50:10 to 5:1 and improve your understanding of mathematical concepts. Remember to avoid common mistakes and practice simplifying ratios to become proficient in this skill.
Take Action!
We encourage you to practice simplifying ratios with different examples and explore real-world applications. Share your experiences and questions in the comments below, and don't forget to share this article with others who may benefit from it.
What is the greatest common divisor (GCD) of 50 and 10?
+The greatest common divisor (GCD) of 50 and 10 is 10.
Why is simplifying ratios important?
+Simplifying ratios helps to reduce complexity, facilitates comparisons, and is essential in various fields, such as science, engineering, and finance.
What are some common mistakes to avoid when simplifying ratios?
+Common mistakes to avoid include failing to find the greatest common divisor (GCD) correctly, not dividing both numbers by the GCD, and making calculation errors.