Expanded Form Of 0.68 With Decimals Explained

Quick Links :

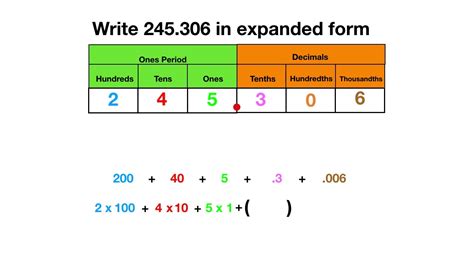
The concept of expanded form with decimals is a fundamental idea in mathematics that helps students understand the place value of digits in a number. In this article, we will delve into the concept of expanded form with decimals, using the number 0.68 as an example. We will explore the benefits of using expanded form, how to convert a decimal number to expanded form, and provide practical examples to reinforce understanding.

What is Expanded Form with Decimals?

Expanded form with decimals is a way of expressing a decimal number in terms of its place value. It involves breaking down the number into its constituent parts, with each part representing a specific place value. In the case of 0.68, the expanded form would show the value of each digit in terms of tenths and hundredths.

Benefits of Using Expanded Form with Decimals

Using expanded form with decimals has several benefits, including:

• Improved understanding of place value: Expanded form helps students understand the concept of place value, which is essential for performing arithmetic operations with decimals.
• Enhanced visualization: Expanded form provides a visual representation of the number, making it easier for students to comprehend the relationships between digits.
• Better accuracy: By breaking down a decimal number into its constituent parts, students can reduce errors when performing calculations.

Converting 0.68 to Expanded Form

To convert 0.68 to expanded form, we need to identify the place value of each digit. In this case, the 6 represents 6 tenths, and the 8 represents 8 hundredths. Therefore, the expanded form of 0.68 is:

0.68 = 6/10 + 8/100

Alternatively, we can express the expanded form using decimal notation:

0.68 = 0.6 + 0.08

Practical Examples of Expanded Form with Decimals

Here are some practical examples of expanded form with decimals:

• 0.45 = 4/10 + 5/100
• 0.27 = 2/10 + 7/100
• 0.93 = 9/10 + 3/100

How to Convert a Decimal Number to Expanded Form

Converting a decimal number to expanded form involves identifying the place value of each digit. Here are the steps:

1. Identify the decimal point and the digits to the right of it.
2. Determine the place value of each digit, starting from the digit immediately to the right of the decimal point.
3. Express each digit in terms of its place value, using fractions or decimal notation.
4. Combine the fractions or decimal values to obtain the expanded form.

Common Misconceptions about Expanded Form with Decimals

One common misconception about expanded form with decimals is that it is only used for simple decimal numbers. However, expanded form can be used for more complex decimal numbers, such as those with multiple digits to the right of the decimal point.

Conclusion

In conclusion, expanded form with decimals is a powerful tool for understanding the place value of digits in a decimal number. By breaking down a decimal number into its constituent parts, students can gain a deeper understanding of the relationships between digits and improve their accuracy when performing arithmetic operations. We hope this article has provided a comprehensive overview of expanded form with decimals, using the number 0.68 as an example.

Call to Action

We encourage readers to share their experiences with expanded form with decimals in the comments section below. How do you use expanded form in your teaching or learning practice? Do you have any tips or resources to share?

FAQ Section