5 Ways To Write Equations In Logarithmic Form

Quick Links :

The world of mathematics is full of fascinating concepts, and logarithms are one of the most powerful tools used to describe the behavior of many natural phenomena. Writing equations in logarithmic form can be a bit tricky, but with the right techniques, you can master this skill. In this article, we will explore five ways to write equations in logarithmic form, along with practical examples and explanations.

Understanding Logarithmic Form

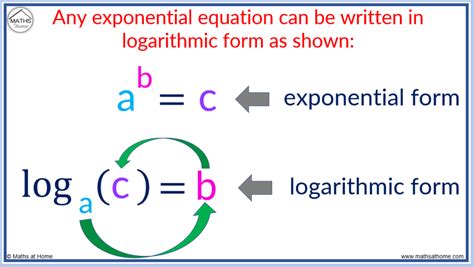
Before we dive into the five ways to write equations in logarithmic form, it's essential to understand what logarithmic form means. Logarithmic form is a way of expressing an exponential equation in a more manageable and interpretable form. In essence, it's a way of solving for the exponent.

Definition of Logarithms

A logarithm is the power to which a base number must be raised to obtain a given value. In mathematical terms, if $x = b^y$, then $y = \log_b x$. Here, $b$ is the base, $x$ is the result, and $y$ is the exponent.

Method 1: Using the Definition of Logarithms

The first method to write equations in logarithmic form is by using the definition of logarithms. Given an exponential equation $x = b^y$, we can rewrite it in logarithmic form as $y = \log_b x$.

Example:

$2^3 = 8$

Using the definition of logarithms, we can rewrite this equation as:

$3 = \log_2 8$

Method 2: Using Logarithmic Properties

The second method is by using logarithmic properties. There are several logarithmic properties, including the product rule, quotient rule, and power rule. These properties can be used to rewrite equations in logarithmic form.

Example:

$\log 2 + \log 3 = \log (2 \cdot 3)$

Using the product rule, we can rewrite this equation as:

$\log 6 = \log 2 + \log 3$

Method 3: Using Exponentials and Logarithms Together

The third method is by using exponentials and logarithms together. This method involves using the fact that exponential and logarithmic functions are inverse functions.

Example:

$e^x = 2$

Using the fact that exponential and logarithmic functions are inverse functions, we can rewrite this equation as:

$x = \ln 2$

Method 4: Using Logarithmic Identities

The fourth method is by using logarithmic identities. There are several logarithmic identities, including the identity $\log_b x = \frac{\log_a x}{\log_a b}$.

Example:

$\log_2 8 = \frac{\log 8}{\log 2}$

Using the logarithmic identity, we can rewrite this equation as:

$3 = \frac{\log 8}{\log 2}$

Method 5: Using Calculator or Software

The fifth and final method is by using a calculator or software. Most calculators and software have built-in logarithmic functions that can be used to rewrite equations in logarithmic form.

Example:

Using a calculator, we can find the logarithm of 8 to the base 2 as:

$\log_2 8 = 3$

In conclusion, writing equations in logarithmic form can be achieved using five different methods: using the definition of logarithms, using logarithmic properties, using exponentials and logarithms together, using logarithmic identities, and using a calculator or software. Each method has its own strengths and weaknesses, and the choice of method depends on the specific problem and the desired outcome.

We hope this article has been informative and helpful in understanding how to write equations in logarithmic form. If you have any questions or need further clarification, please don't hesitate to ask. Share your thoughts and experiences in the comments section below.