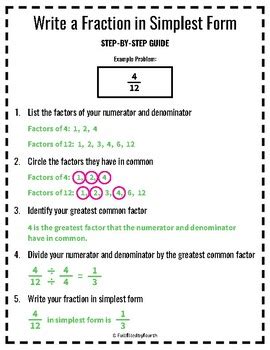
The decimal number 0.65 can be converted into a fraction in simplest form. To do this, we can write 0.65 as a fraction with a denominator of 100, since there are two decimal places.
0.65 = 65/100
We can simplify this fraction by dividing both the numerator and the denominator by 5.
65 ÷ 5 = 13 100 ÷ 5 = 20
So, the simplified fraction is:
0.65 = 13/20
Therefore, the decimal number 0.65 as a fraction in simplest form is 13/20.
What is a Fraction?
A fraction is a way of expressing a part of a whole as a ratio of two numbers. It consists of a numerator (the top number) and a denominator (the bottom number). The numerator tells us how many equal parts we have, and the denominator tells us how many parts the whole is divided into.
Why is it Important to Simplify Fractions?
Simplifying fractions is important because it helps us to express the fraction in its most basic form, making it easier to understand and work with. Simplifying fractions also helps to avoid confusion and errors when adding, subtracting, multiplying, or dividing fractions.
How to Simplify Fractions
To simplify a fraction, we need to find the greatest common divisor (GCD) of the numerator and the denominator. The GCD is the largest number that divides both numbers without leaving a remainder. Once we have found the GCD, we can divide both the numerator and the denominator by the GCD to simplify the fraction.

For example, let's simplify the fraction 12/18.
- Find the GCD of 12 and 18, which is 6.
- Divide both the numerator and the denominator by the GCD: 12 ÷ 6 = 2 and 18 ÷ 6 = 3.
- Write the simplified fraction: 2/3.
Types of Fractions
There are several types of fractions, including:
- Proper fractions: These are fractions where the numerator is less than the denominator. Examples include 1/2, 3/4, and 2/3.
- Improper fractions: These are fractions where the numerator is greater than the denominator. Examples include 3/2, 5/3, and 7/4.
- Mixed numbers: These are fractions that consist of a whole number and a proper fraction. Examples include 2 1/2, 3 3/4, and 1 1/3.
Real-World Applications of Fractions
Fractions are used in many real-world applications, including:
- Cooking: Recipes often require fractions of ingredients, such as 1/4 cup of sugar or 3/4 teaspoon of salt.
- Music: Music is based on fractions, with rhythms and time signatures expressed as fractions of a beat.
- Science: Fractions are used to express measurements and quantities in science, such as 3/4 of a liter or 2/3 of a meter.

In conclusion, fractions are an essential part of mathematics and are used in many real-world applications. Simplifying fractions is important to express them in their most basic form and to avoid confusion and errors.
How to Convert Decimals to Fractions
Converting decimals to fractions is a simple process that involves writing the decimal as a fraction with a denominator of 10, 100, or 1000, depending on the number of decimal places.
- Decimals with one decimal place: Write the decimal as a fraction with a denominator of 10. For example, 0.5 = 5/10 = 1/2.
- Decimals with two decimal places: Write the decimal as a fraction with a denominator of 100. For example, 0.25 = 25/100 = 1/4.
- Decimals with three decimal places: Write the decimal as a fraction with a denominator of 1000. For example, 0.125 = 125/1000 = 1/8.

FAQ Section:
What is a fraction?
+A fraction is a way of expressing a part of a whole as a ratio of two numbers.
Why is it important to simplify fractions?
+Simplifying fractions helps to express the fraction in its most basic form, making it easier to understand and work with.
How do I convert decimals to fractions?
+To convert decimals to fractions, write the decimal as a fraction with a denominator of 10, 100, or 1000, depending on the number of decimal places.