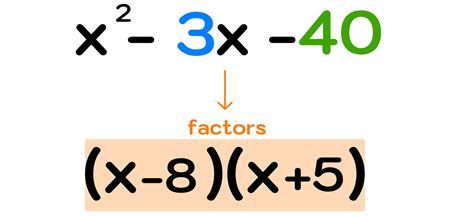Understanding the factored form of a mathematical expression is a fundamental concept in algebra and mathematics. The factored form helps in simplifying complex expressions, solving equations, and finding the roots of a polynomial. However, many students struggle to factorize expressions, and it becomes a daunting task for them. But, with a few simple steps and some practice, anyone can master the art of factoring. In this article, we will unlock the factored form in 3 easy steps, making it easier for you to tackle even the most complex expressions.

Step 1: Identify the Type of Expression
The first step in unlocking the factored form is to identify the type of expression you are dealing with. There are several types of expressions, including:
- Monomial: A single term expression, such as 2x or 3y.
- Binomial: A two-term expression, such as x + 3 or 2x - 4.
- Trinomial: A three-term expression, such as x^2 + 5x + 6 or 2x^2 - 7x - 3.
- Polynomial: A multi-term expression, such as x^3 + 2x^2 - 7x - 12.
Identifying the type of expression will help you determine the best approach to factorize it.
Example: Identify the Type of Expression
Suppose we have the expression x^2 + 4x + 4. This is a trinomial expression, as it has three terms.
Step 2: Look for Common Factors
Once you have identified the type of expression, the next step is to look for common factors. Common factors are terms that appear in every term of the expression. By factoring out the common factors, you can simplify the expression and make it easier to work with.

Example: Look for Common Factors
Using the same example, x^2 + 4x + 4, we can see that there is a common factor of 4 in every term.
x^2 + 4x + 4 = 4(x^2/4 + x + 1)
By factoring out the common factor of 4, we have simplified the expression and made it easier to work with.
Step 3: Factorize Using Algebraic Identities
The final step in unlocking the factored form is to factorize the expression using algebraic identities. Algebraic identities are mathematical formulas that help us factorize expressions. Some common algebraic identities include:
- Difference of Squares: a^2 - b^2 = (a + b)(a - b)
- Sum and Difference of Cubes: a^3 + b^3 = (a + b)(a^2 - ab + b^2) and a^3 - b^3 = (a - b)(a^2 + ab + b^2)
- Perfect Square Trinomial: a^2 + 2ab + b^2 = (a + b)^2

Example: Factorize Using Algebraic Identities
Using the same example, x^2 + 4x + 4, we can factorize it using the perfect square trinomial identity.
x^2 + 4x + 4 = (x + 2)^2
By applying the algebraic identity, we have factored the expression and found the factored form.
Now that we have unlocked the factored form in 3 easy steps, you can apply these steps to any expression and simplify it. Remember to identify the type of expression, look for common factors, and factorize using algebraic identities.

Real-World Applications of Factored Form
The factored form has numerous real-world applications in mathematics, science, and engineering. Some examples include:
- Solving Quadratic Equations: The factored form is used to solve quadratic equations, which are used to model real-world problems such as projectile motion and electrical circuits.
- Finding Roots of Polynomials: The factored form is used to find the roots of polynomials, which is essential in algebra and calculus.
- Simplifying Complex Expressions: The factored form is used to simplify complex expressions, making it easier to analyze and solve problems.

Conclusion
In conclusion, the factored form is an essential concept in mathematics that helps us simplify complex expressions, solve equations, and find the roots of polynomials. By following the 3 easy steps outlined in this article, you can unlock the factored form and apply it to various real-world problems. Remember to practice regularly and use online resources to reinforce your understanding of the factored form.

Call to Action
We hope this article has helped you understand the concept of factored form and its applications. If you have any questions or need further clarification, please don't hesitate to ask. Share your thoughts and experiences in the comments section below, and don't forget to like and share this article with your friends and colleagues.

What is the factored form of an expression?
+The factored form of an expression is a way of writing the expression as a product of simpler expressions, called factors.
How do I identify the type of expression?
+You can identify the type of expression by counting the number of terms it has. If it has one term, it's a monomial. If it has two terms, it's a binomial. If it has three terms, it's a trinomial. If it has more than three terms, it's a polynomial.
What are some common algebraic identities used for factoring?
+Some common algebraic identities used for factoring include the difference of squares, sum and difference of cubes, and perfect square trinomial.