Factor X^3 + 1 Easily In 3 Simple Steps

Quick Links :

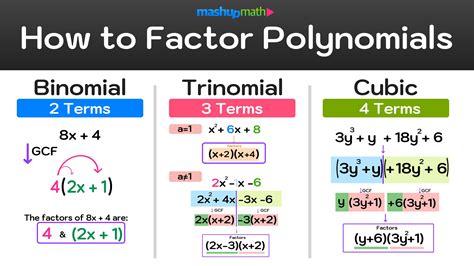
The cubic polynomial X^3 + 1 may seem daunting to factor, but it can be easily broken down using a few simple steps. Understanding how to factor this polynomial can be incredibly useful in various mathematical and scientific applications.

Factor X^3 + 1 can be viewed as a sum of cubes, which is a special algebraic expression that can be factored using a specific formula. Recognizing this pattern is crucial to simplifying the polynomial.

Step 1: Identify the Sum of Cubes Pattern

The first step in factoring X^3 + 1 is to recognize that it follows the sum of cubes pattern. This pattern can be represented by the formula a^3 + b^3, where 'a' and 'b' are constants or variables.

In the case of X^3 + 1, 'a' is X and 'b' is 1. By identifying this pattern, we can apply the sum of cubes formula to factor the polynomial.

Sum of Cubes Formula

The sum of cubes formula is:

a^3 + b^3 = (a + b)(a^2 - ab + b^2)

This formula allows us to factor the polynomial into the product of two binomials.

Step 2: Apply the Sum of Cubes Formula

Now that we have identified the sum of cubes pattern and the formula, we can apply it to factor X^3 + 1. Substituting 'a' as X and 'b' as 1 into the formula, we get:

X^3 + 1 = (X + 1)(X^2 - X*1 + 1^2)

Simplifying the expression inside the parentheses, we get:

X^3 + 1 = (X + 1)(X^2 - X + 1)

Step 3: Final Factorization

We have now factored X^3 + 1 into the product of two binomials using the sum of cubes formula. The final factorization is:

X^3 + 1 = (X + 1)(X^2 - X + 1)

This factorization can be used in various mathematical and scientific applications, such as solving equations and finding roots.

By following these three simple steps, we can easily factor X^3 + 1 and break down the polynomial into its constituent parts.

Practical Applications

Understanding how to factor X^3 + 1 can be incredibly useful in various practical applications, such as:

• Solving equations: Factoring X^3 + 1 can help us solve equations involving this polynomial.
• Finding roots: The factorization of X^3 + 1 can be used to find the roots of the polynomial.
• Algebraic manipulations: The factorization of X^3 + 1 can be used to simplify algebraic expressions and manipulate equations.

By mastering the art of factoring X^3 + 1, we can unlock a wide range of mathematical and scientific possibilities.

We hope this article has been informative and helpful in your understanding of how to factor X^3 + 1. If you have any questions or need further clarification, please don't hesitate to ask.

What are your thoughts on factoring X^3 + 1? Share your experiences and tips in the comments below!