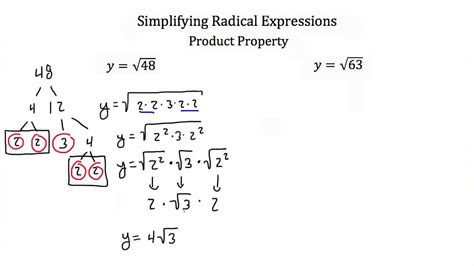
Simplifying Radical Expressions: 3p3q 34

Quick Links :

Simplifying radical expressions is an essential concept in mathematics, particularly in algebra and geometry. Radical expressions involve the use of roots, such as square roots, cube roots, and so on. These expressions can be simplified to make them easier to work with and understand.

What is a Radical Expression?

A radical expression is a mathematical expression that contains a root, such as a square root or cube root. The root is denoted by a radical sign, which looks like a check mark (√). For example, √(x + 1) is a radical expression.

Why Simplify Radical Expressions?

Simplifying radical expressions is important because it helps to:

• Make the expression easier to read and understand
• Simplify calculations and solve equations
• Identify equivalent expressions
• Make it easier to compare and order expressions

Basic Rules for Simplifying Radical Expressions

To simplify radical expressions, we need to follow some basic rules:

• The radicand (the expression inside the radical sign) must be non-negative.
• The index (the number outside the radical sign) must be a positive integer.
• The radical sign can only be removed if the index is an even number.

Rule 1: Simplify the Radicand

The first step in simplifying a radical expression is to simplify the radicand. This involves factoring out any perfect squares or perfect cubes from the radicand.

For example, √(4x + 4) = √(4(x + 1)) = 2√(x + 1)

Rule 2: Simplify the Index

The next step is to simplify the index. If the index is an even number, we can remove the radical sign and take the root of the radicand.

For example, √(x^2) = x

Rule 3: Use the Product Rule

The product rule states that the product of two radical expressions is equal to the product of the radicands.

For example, √(x) * √(y) = √(xy)

Rule 4: Use the Quotient Rule

The quotient rule states that the quotient of two radical expressions is equal to the quotient of the radicands.

For example, √(x) / √(y) = √(x/y)

Simplifying Radical Expressions with Variables

Simplifying radical expressions with variables involves applying the same rules as before, but with variables instead of numbers.

For example, √(3x + 2) = √(3(x + 2/3)) = √3√(x + 2/3)

Example 1: Simplify the Expression √(x^2 + 4x + 4)

Using the product rule, we can simplify the expression as follows:

√(x^2 + 4x + 4) = √((x + 2)^2) = x + 2

Example 2: Simplify the Expression √(9x^2 - 16)

Using the difference of squares formula, we can simplify the expression as follows:

√(9x^2 - 16) = √((3x)^2 - 4^2) = √((3x + 4)(3x - 4)) = √(3x + 4)√(3x - 4)

Conclusion

Simplifying radical expressions is an essential skill in mathematics, and it requires a good understanding of the basic rules and properties of radicals. By applying these rules and using the product and quotient rules, we can simplify even the most complex radical expressions.