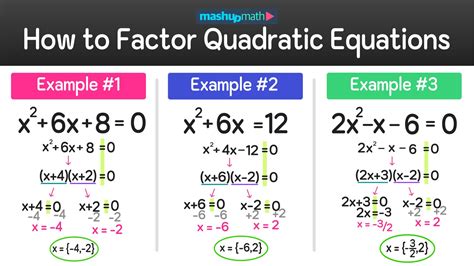
The world of algebra can be a complex and fascinating place, especially when it comes to quadratic expressions. Quadratic expressions are polynomial expressions that contain a squared variable, and they play a crucial role in various mathematical and real-world applications. In this article, we will delve into the world of factoring quadratic expressions, with a specific focus on the expression x2 - 16xy + 64y2.
The Importance of Factoring Quadratic Expressions
Factoring quadratic expressions is a fundamental concept in algebra, and it has numerous applications in mathematics, physics, engineering, and other fields. By factoring a quadratic expression, we can simplify complex equations, solve quadratic equations, and gain insight into the underlying structure of the expression. In this article, we will explore the benefits of factoring quadratic expressions and provide a step-by-step guide on how to factor the expression x2 - 16xy + 64y2.

Understanding the Structure of Quadratic Expressions
Before we dive into factoring, let's take a closer look at the structure of quadratic expressions. A quadratic expression is a polynomial expression that contains a squared variable, and it can be written in the general form:
ax2 + bx + c
where a, b, and c are constants, and x is the variable. The expression x2 - 16xy + 64y2 is a quadratic expression in two variables, x and y.
Types of Quadratic Expressions
Quadratic expressions can be classified into several types, including:
- Monic quadratic expressions: These are quadratic expressions where the coefficient of the squared term is 1. For example, x2 + 5x + 6 is a monic quadratic expression.
- Non-monic quadratic expressions: These are quadratic expressions where the coefficient of the squared term is not 1. For example, 2x2 + 3x + 1 is a non-monic quadratic expression.
- Perfect square trinomials: These are quadratic expressions that can be written as the square of a binomial. For example, x2 + 2xy + y2 is a perfect square trinomial.
Factoring Quadratic Expressions: x2 - 16xy + 64y2
Now that we have a basic understanding of quadratic expressions, let's move on to factoring the expression x2 - 16xy + 64y2. This expression is a perfect square trinomial, and it can be factored as follows:
x2 - 16xy + 64y2 = (x - 8y)2
To factor this expression, we need to find two binomials whose product is equal to the original expression. In this case, the two binomials are (x - 8y) and (x - 8y).

Step-by-Step Guide to Factoring Quadratic Expressions
Factoring quadratic expressions can be a challenging task, but it can be made easier by following a step-by-step guide. Here's a step-by-step guide to factoring quadratic expressions:
- Write the expression in standard form: Write the quadratic expression in the standard form ax2 + bx + c.
- Look for common factors: Look for common factors among the terms of the expression.
- Check if the expression is a perfect square trinomial: Check if the expression is a perfect square trinomial by looking for two binomials whose product is equal to the original expression.
- Use the quadratic formula: If the expression is not a perfect square trinomial, use the quadratic formula to factor the expression.
The Quadratic Formula
The quadratic formula is a powerful tool for factoring quadratic expressions. The quadratic formula is given by:
x = (-b ± √(b2 - 4ac)) / 2a
This formula can be used to factor quadratic expressions by finding the roots of the equation.

Real-World Applications of Factoring Quadratic Expressions
Factoring quadratic expressions has numerous real-world applications in mathematics, physics, engineering, and other fields. Some examples of real-world applications include:
- Projectile motion: Factoring quadratic expressions is used to model the trajectory of projectiles in physics.
- Optimization problems: Factoring quadratic expressions is used to solve optimization problems in mathematics and engineering.
- Computer graphics: Factoring quadratic expressions is used to create 3D models and animations in computer graphics.

Conclusion
Factoring quadratic expressions is a fundamental concept in algebra, and it has numerous applications in mathematics, physics, engineering, and other fields. In this article, we have explored the benefits of factoring quadratic expressions and provided a step-by-step guide on how to factor the expression x2 - 16xy + 64y2. We have also discussed the quadratic formula and its applications in factoring quadratic expressions.

What is a quadratic expression?
+A quadratic expression is a polynomial expression that contains a squared variable.
What is the quadratic formula?
+The quadratic formula is a formula used to solve quadratic equations. It is given by x = (-b ± √(b2 - 4ac)) / 2a.
What are some real-world applications of factoring quadratic expressions?
+Factoring quadratic expressions has numerous real-world applications in mathematics, physics, engineering, and other fields, including projectile motion, optimization problems, and computer graphics.
We hope this article has been informative and helpful in your understanding of factoring quadratic expressions. If you have any further questions or comments, please don't hesitate to ask.