Simplify Radicals In 3 Easy Steps

Quick Links :

Hide

Simplify Radicals In 3 Easy Steps

Radicals, also known as roots, are a fundamental concept in mathematics, particularly in algebra and geometry. Simplifying radicals is an essential skill that can help you solve complex mathematical problems with ease. In this article, we will show you how to simplify radicals in 3 easy steps.

Radicals are expressed as √x, where x is the radicand. Simplifying radicals involves breaking down the radicand into its prime factors and then extracting the square root. This process can be challenging, but with the right approach, you can simplify radicals with confidence.

Step 1: Factor the Radicand

The first step in simplifying radicals is to factor the radicand into its prime factors. This involves breaking down the radicand into its simplest building blocks. For example, if the radicand is 12, we can factor it as 2 × 2 × 3.

To factor the radicand, you can use the following methods:

• Prime factorization: Break down the radicand into its prime factors using the prime factorization method.
• Greatest common factor (GCF): Find the greatest common factor of the radicand and its factors.

Step 2: Identify Perfect Squares

The second step in simplifying radicals is to identify perfect squares within the radicand. Perfect squares are numbers that can be expressed as the square of an integer, such as 4, 9, or 16.

To identify perfect squares, you can use the following methods:

• Look for numbers that are perfect squares, such as 4, 9, or 16.
• Check if the radicand is a perfect square by taking the square root of the number and checking if it is an integer.

Step 3: Simplify the Radical

The final step in simplifying radicals is to simplify the radical by extracting the square root. This involves dividing the radicand by the perfect square and then taking the square root of the remaining number.

For example, if we have the radical √12, we can simplify it by factoring the radicand as 2 × 2 × 3, identifying the perfect square 4, and then simplifying the radical as √(4 × 3) = 2√3.

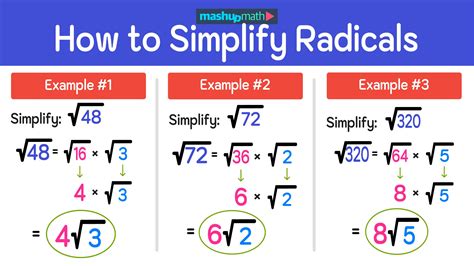
Examples of Simplifying Radicals

Here are some examples of simplifying radicals:

• √12 = √(4 × 3) = 2√3
• √48 = √(16 × 3) = 4√3
• √75 = √(25 × 3) = 5√3

Benefits of Simplifying Radicals

Simplifying radicals has several benefits, including:

• Simplifying complex mathematical expressions
• Making it easier to solve equations and inequalities
• Improving mathematical accuracy and precision

Common Mistakes to Avoid When Simplifying Radicals

Here are some common mistakes to avoid when simplifying radicals:

• Forgetting to factor the radicand
• Not identifying perfect squares
• Simplifying the radical incorrectly

Practical Applications of Simplifying Radicals

Simplifying radicals has several practical applications, including:

• Physics and engineering: Simplifying radicals is used to solve problems involving distance, velocity, and acceleration.
• Computer science: Simplifying radicals is used in algorithms and data structures.
• Economics: Simplifying radicals is used to model economic systems and make predictions.

Conclusion

Simplifying radicals is an essential skill in mathematics that can help you solve complex problems with ease. By following the 3 easy steps outlined in this article, you can simplify radicals with confidence. Remember to factor the radicand, identify perfect squares, and simplify the radical. With practice and patience, you can master the art of simplifying radicals.