Simplifying Fractions: What Is Simplest Exact Form

Quick Links :

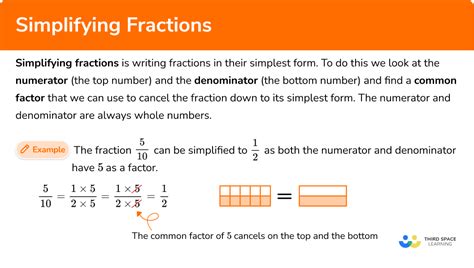
In mathematics, fractions are a fundamental concept used to represent a part of a whole. However, working with fractions can be complex, especially when dealing with large numbers or multiple fractions. To simplify calculations and comparisons, fractions need to be expressed in their simplest exact form. But what is simplest exact form, and how do we achieve it?

Fractions are used to represent a portion of a whole, and they consist of two parts: the numerator (the top number) and the denominator (the bottom number). For instance, in the fraction 3/4, 3 is the numerator, and 4 is the denominator. When we simplify fractions, we aim to find an equivalent fraction with a smaller numerator and denominator while maintaining the same value.

Why Simplify Fractions?

Simplifying fractions has several benefits. It makes calculations easier, reduces errors, and facilitates comparisons between fractions. Imagine trying to add 1/6 and 1/8; if you don't simplify the fractions first, the calculation becomes unnecessarily complicated. By expressing fractions in their simplest form, you can perform arithmetic operations more efficiently and accurately.

How to Simplify Fractions

To simplify a fraction, you need to find the greatest common divisor (GCD) of the numerator and the denominator. The GCD is the largest number that divides both numbers without leaving a remainder. Once you have the GCD, you can divide both the numerator and the denominator by this number to obtain the simplified fraction.

For example, let's simplify the fraction 12/18:

1. Find the GCD of 12 and 18: GCD(12, 18) = 6
2. Divide both the numerator and the denominator by the GCD: 12 ÷ 6 = 2, 18 ÷ 6 = 3
3. Write the simplified fraction: 2/3

Simplifying Fractions with Variables

When working with fractions that contain variables, the process of simplification is similar, but you need to consider the variables as well. To simplify a fraction with variables, factor out the greatest common factor (GCF) from the numerator and the denominator.

For instance, simplify the fraction (2x + 4)/(4x + 8):

1. Factor out the GCF: 2( x + 2 ) / 4( x + 2 )
2. Cancel out the common factor ( x + 2 ): 2/4
3. Simplify the fraction: 1/2

Simplifying Complex Fractions

Complex fractions, also known as compound fractions, are fractions that contain other fractions within them. To simplify complex fractions, follow these steps:

1. Simplify the inner fractions, if possible.
2. Find the least common denominator (LCD) of the inner fractions.
3. Multiply the inner fractions by the necessary multiples to obtain the LCD.
4. Simplify the resulting fraction.

For example, simplify the complex fraction (1/2 + 1/4) / (3/4 - 1/2):

1. Simplify the inner fractions: (1/2 + 1/4) = 3/4, (3/4 - 1/2) = 1/4
2. Find the LCD: LCD(3/4, 1/4) = 4
3. Multiply the inner fractions by the necessary multiples: (3/4) / (1/4) = 3
4. Simplify the resulting fraction: 3

Real-World Applications of Simplifying Fractions

Simplifying fractions has numerous real-world applications, including:

1. Cooking: When scaling recipes up or down, you need to simplify fractions to maintain the correct proportions.
2. Finance: Simplifying fractions is essential for calculating interest rates, investment returns, and other financial metrics.
3. Science: Fractions are used to express scientific measurements, such as density and concentration. Simplifying these fractions helps scientists compare and analyze data.
4. Music: Musicians use fractions to represent rhythm and timing. Simplifying these fractions enables them to create complex musical patterns.

Common Mistakes When Simplifying Fractions

When simplifying fractions, it's essential to avoid common mistakes that can lead to incorrect results:

1. Not finding the GCD: Failing to find the greatest common divisor can result in an incorrect simplified fraction.
2. Canceling out the wrong factors: Be careful when canceling out common factors to avoid simplifying the fraction incorrectly.
3. Not considering variables: When working with fractions that contain variables, make sure to consider the variables when simplifying the fraction.

By following the steps outlined above and avoiding common mistakes, you can simplify fractions with confidence and accuracy.

Conclusion

Simplifying fractions is an essential skill in mathematics, and it has numerous real-world applications. By understanding the importance of simplifying fractions and mastering the techniques outlined above, you can improve your mathematical skills and apply them to a wide range of problems. Remember to always find the GCD, consider variables, and avoid common mistakes to ensure accurate results.

We hope this article has helped you understand the concept of simplest exact form and how to simplify fractions. Do you have any questions or topics you'd like us to explore further? Share your thoughts in the comments below, and don't forget to share this article with others who might find it helpful.